Advertisements
Advertisements
प्रश्न
Find all zeroes of the polynomial `(2x^4 - 9x^3 + 5x^2 + 3x - 1)` if two of its zeroes are `(2 + sqrt3)` and `(2 - sqrt3)`
उत्तर
It is given that `2 + sqrt3` and `2 - sqrt3` are two zeroes of the polynomial f(x) = `24^4 - 9x^3 + 5x^2 + 3x - 1`
`:. {x - (2 + sqrt3)} {x - (2-sqrt3)} = (x - 2 - sqrt3) (x - 2 + sqrt3)`
` = (x - 2)^2 - (sqrt3)^2`
`= x^2 - 4x + 4 - 3`
`= x^2 - 4x + 1`
is a factor of f(x)
Now divide f(x) by `x^2 - 4x + 1`
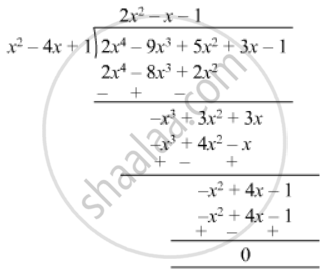
`:, f(x) = (x^2 - 4x + 1)(2x^2 - x - 1)`
Hence, other two zeroes of f(x) are the zeroes of the polynomial `2x^2 - x - 1`
`2x^2 - x - 1 = 2x^2 - 2x + x - 1 = 2x(x - 1)+ 1(x - 1) = (2x + 1) (x - 1)``
Hence the other two zeroes are `-1/2` and 1
APPEARS IN
संबंधित प्रश्न
Find a quadratic polynomial with the given numbers as the sum and product of its zeroes respectively.
`0, sqrt5`
If α and β are the zeros of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate α2β + αβ2
Find the zeroes of the quadratic polynomial `2x^2 ˗ 11x + 15` and verify the relation between the zeroes and the coefficients.
Find the zeroes of the quadratic polynomial `(8x^2 ˗ 4)` and verify the relation between the zeroes and the coefficients
Find the zeroes of the quadratic polynomial `(5y^2 + 10y)` and verify the relation between the zeroes and the coefficients.
What should be subtracted to the polynomial x2 − 16x + 30, so that 15 is the zero of the resulting polynomial?
Check whether g(x) is a factor of p(x) by dividing polynomial p(x) by polynomial g(x),
where p(x) = x5 − 4x3 + x2 + 3x +1, g(x) = x3 − 3x + 1
If one of the zeroes of the quadratic polynomial (k – 1)x2 + k x + 1 is –3, then the value of k is ______.
Find the zeroes of the following polynomials by factorisation method and verify the relations between the zeroes and the coefficients of the polynomials:
t3 – 2t2 – 15t
For the following, find a quadratic polynomial whose sum and product respectively of the zeroes are as given. Also find the zeroes of these polynomials by factorisation.
`21/8, 5/16`
