Advertisements
Advertisements
प्रश्न
Find the coordinate of a point P which divides the line segment joining :
5(2, 6) and R(9, -8) in the ratio 3: 4.
उत्तर
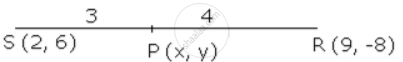
Let the point P divides the line segment SR in the ratio 3:4.
∴ coordinates of P are
x = `(3 xx 9 + 4 xx 2)/(3 + 4) = 5`
y = `(3 xx -8 + 4 xx 6)/(3 + 4) = 0`
APPEARS IN
संबंधित प्रश्न
Determine the ratio in which the line 3x + y – 9 = 0 divides the segment joining the points (1, 3) and (2, 7)
If the points A (6, 1), B (8, 2), C(9, 4) and D(p, 3) are vertices of a parallelogram, taken in order, find the value of p
Find the ratio in which the line segment joining the points (-3, 10) and (6, -8) is divided by (-1, 6).
Find the coordinates of a point A, where AB is the diameter of circle whose centre is (2, -3) and B is (1, 4).
The mid point of the line segment joining (4a, 2b – 3) and (−4, 3b) is (2, –2a). Find the values of a and b.
Find the ratio in which the y-axis divides the line segment joining the points (−4, − 6) and (10, 12). Also find the coordinates of the point of division ?
In what ratio does the point (1, a) divided the join of (−1, 4) and (4, −1) Also, find the value of a.
Show that the lines x = O and y = O trisect the line segment formed by joining the points (-10, -4) and (5, 8). Find the points of trisection.
Using section formula, show that the points A(7, −5), B(9, −3) and C(13, 1) are collinear
If point P(1, 1) divide segment joining point A and point B(–1, –1) in the ratio 5 : 2, then the coordinates of A are ______
