Question

Show that the lines x = O and y = O trisect the line segment formed by joining the points (-10, -4) and (5, 8). Find the points of trisection. 

Answer 1

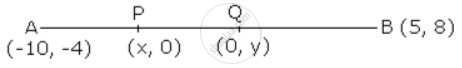
Let P (x, 0) lies on the line y = 0 i.e. x-axis and divides the line segment AB in the ratio k: 1.

Coordinates of P are, 

P (x , 0) = P `((5"k" - 10)/("k" + 1) , (8"k" - 4)/("k" + 1))`

`=> 0 = (8"k" - 4)/("k" + 1) ,  (5"k" - 10)/("k" + 1) = "x"`

`=> 8"k" = 4  , (5(1/2) - 10)/(1/2 + "x") = "x"`  ........from (1)

`=> "k" = 1/2`  ........(1) , x = -5

Hence P(-5, 0) divides AB in the ratio 1: 2. 

Let Q (0, y) lies on the line x = 0 i.e. y - axis and 

divides the line segment AB in the ratio k₁ : 1. 

Coordinates of Q are

Q (0 , y) = Q `((5"k"_1 - 10)/("k"_1 + 1) , (8"k"_1 - 4)/("k"_1 + 1))`

`0 = (5"k"_1 - 10)/("k"_1 + 1) ,  "y" = (8"k"_1 - 4)/("k"_1 + 1)`

`=> 5 "k"_1 = 10  , "y" = (8(2) - 4)/(2 + 1)`   .......from (2)

⇒ k₁ = 2      .... (2)             y = 4

Hence, Q(O, 4) divides in the ratio 2 : 1.

Hence proved Paid Qare the points of trisection of AB.