Advertisements
Advertisements
प्रश्न
Show that the line segment joining the points (-3, 10) and (6, -5) is trisected by the coordinates axis.
उत्तर
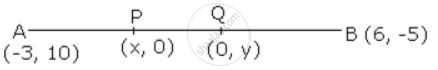
Let the coordinates of two points x-axis and y-axis be P (x, O) and G (0, y) respectively.
Let P divides AB in the ratio k : 1.
Coordinates of P are
P (x , 0) = P `((6"k" - 3)/("k" + 1) , (- 5 "k" + 10)/("k" + 1))`
⇒ 0 = `(- 5 "k" + 10)/("k" + 1)`
⇒ 5 k = 10
⇒ k = 2
Hence P divides AB in the ratio 2: 1.
Let Q divides AB in the ratio k1 : 1.
Coordinates of Q are,
Q (0 , y) = Q `((6"k"_1 - 3)/("k" + 1) , (-5"k" + 10)/("k" + 1))`
`=> 0 = (6"k"_1 - 3)/("k" + 1)`
⇒ 6k1 = 3
⇒ `"k"_1 = 1/2`
Hence Q divides AB in the ratio 1: 2
Hence proved, P and Q are the points of trisection.
APPEARS IN
संबंधित प्रश्न
Prove that (4, – 1), (6, 0), (7, 2) and (5, 1) are the vertices of a rhombus. Is it a square?
If the points A (6, 1), B (8, 2), C (9, 4) and D (k, p) are the vertices of a parallelogram taken in order, then find the values of k and p.
The line segment joining A (2, 3) and B (6, –5) is intercepted by x-axis at the point K. Write down the ordinate of the point K. Hence, find the ratio in which K divides AB. Also, find the coordinates of the point K.
Find the length of the hypotenuse of a square whose side is 16 cm.
M and N are two points on the X axis and Y axis respectively. P (3, 2) divides the line segment MN in the ratio 2 : 3.
Find:
(i) the coordinates of M and N
(ii) slope of the line MN.
Find the ratio in which the line y = -1 divides the line segment joining (6, 5) and (-2, -11). Find the coordinates of the point of intersection.
If point P divides segment AB in the ratio 1 : 3 where A(– 5, 3) and B(3, – 5), then the coordinates of P are ______
Find the ratio in which Y-axis divides the point A(3, 5) and point B(– 6, 7). Find the coordinates of the point
The point which divides the line segment joining the points (7, –6) and (3, 4) in ratio 1 : 2 internally lies in the ______.
If the points A(2, 3), B(–5, 6), C(6, 7) and D(p, 4) are the vertices of a parallelogram ABCD, find the value of p.
