Advertisements
Advertisements
प्रश्न
Find the ratio in which Y-axis divides the point A(3, 5) and point B(– 6, 7). Find the coordinates of the point
उत्तर
Let C be a point on Y-axis which divides seg AB in the ratio m : n
Point C lies on the Y-axis.
∴ its X-coordinate is 0.
Let C = (0, y)
Here,
A(x1, y1) = A(3, 5)
B(x2, y2) = B(– 6, 7)
By Section formula,
x = `("m"x_2 + "n"x_1)/("m" + "n")`
∴ 0 = `(-6"m" + 3"n")/("m" + "n")`
∴ – 6m + 3n = 0
∴ 3n = 6m
∴ `"m"/"n" = 3/6`
∴ `"m"/"n" = 1/2` ......(i)
∴ m : n = 1 : 2
By section formula,
y = `("m"y_2 + "n"y_1)/("m" + "n")`
y = `(7"m" + 5"n")/("m" + "n")`
= `(7"m" + 5(2"m"))/("m" + 2"m")` ......[From (i), n = 2m]
= `(7"m" + 10"m")/(3"m")`
= `(17"m")/(3"m")`
∴ Y-axis divides the seg AB in the ratio 1 : 2 and the co-ordinates of that point is `(0, 17/3)`.
APPEARS IN
संबंधित प्रश्न
Prove that (4, – 1), (6, 0), (7, 2) and (5, 1) are the vertices of a rhombus. Is it a square?
Find the coordinates of the points of trisection of the line segment joining (4, -1) and (-2, -3).
Find the area of a rhombus if its vertices are (3, 0), (4, 5), (− 1, 4) and (− 2, −1) taken in order.
[Hint: Area of a rhombus = `1/2` (product of its diagonals)]
Show that the mid-point of the line segment joining the points (5, 7) and (3, 9) is also the mid-point of the line segment joining the points (8, 6) and (0, 10).
In what ratio does the point (a, 6) divide the join of (–4, 3) and (2, 8)? Also, find the value of a.
The line segment joining the points M(5, 7) and N(–3, 2) is intersected by the y-axis at point L. Write down the abscissa of L. Hence, find the ratio in which L divides MN. Also, find the co-ordinates of L.
The line segment joining A (2, 3) and B (6, –5) is intercepted by x-axis at the point K. Write down the ordinate of the point K. Hence, find the ratio in which K divides AB. Also, find the coordinates of the point K.
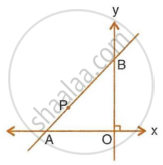
In the given figure, line APB meets the x-axis at point A and y-axis at point B. P is the point (−4, 2) and AP : PB = 1 : 2. Find the co-ordinates of A and B.
A(20, 0) and B(10, –20) are two fixed points. Find the co-ordinates of the point P in AB such that : 3PB = AB. Also, find the co-ordinates of some other point Q in AB such that : AB = 6 AQ.
In what ratio is the line joining (2, -1) and (-5, 6) divided by the y axis ?
Using section formula, show that the points A(7, −5), B(9, −3) and C(13, 1) are collinear
If point P(1, 1) divide segment joining point A and point B(–1, –1) in the ratio 5 : 2, then the coordinates of A are ______
The fourth vertex D of a parallelogram ABCD, whose three vertices are A(–2, 3), B(6, 7) and C(8, 3), is ______.
Find the ratio in which the point `P(3/4, 5/12)` divides the line segment joining the points `A(1/2, 3/2)` and B(2, –5).
The line segment joining the points A(3, 2) and B(5, 1) is divided at the point P in the ratio 1 : 2 and it lies on the line 3x – 18y + k = 0. Find the value of k.
Find the ratio in which the line 2x + 3y – 5 = 0 divides the line segment joining the points (8, –9) and (2, 1). Also find the coordinates of the point of division.
Find the co-ordinates of the points of trisection of the line segment joining the points (5, 3) and (4, 5).
What is the ratio in which the line segment joining (2, -3) and (5, 6) is divided by x-axis?
