Advertisements
Advertisements
Question
Show that the line segment joining the points (-3, 10) and (6, -5) is trisected by the coordinates axis.
Solution
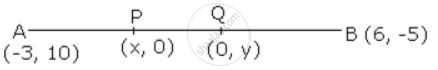
Let the coordinates of two points x-axis and y-axis be P (x, O) and G (0, y) respectively.
Let P divides AB in the ratio k : 1.
Coordinates of P are
P (x , 0) = P `((6"k" - 3)/("k" + 1) , (- 5 "k" + 10)/("k" + 1))`
⇒ 0 = `(- 5 "k" + 10)/("k" + 1)`
⇒ 5 k = 10
⇒ k = 2
Hence P divides AB in the ratio 2: 1.
Let Q divides AB in the ratio k1 : 1.
Coordinates of Q are,
Q (0 , y) = Q `((6"k"_1 - 3)/("k" + 1) , (-5"k" + 10)/("k" + 1))`
`=> 0 = (6"k"_1 - 3)/("k" + 1)`
⇒ 6k1 = 3
⇒ `"k"_1 = 1/2`
Hence Q divides AB in the ratio 1: 2
Hence proved, P and Q are the points of trisection.
APPEARS IN
RELATED QUESTIONS
If A (5, –1), B(–3, –2) and C(–1, 8) are the vertices of triangle ABC, find the length of median through A and the coordinates of the centroid.
Find the ratio in which P(4, m) divides the line segment joining the points A(2, 3) and B(6, –3). Hence find m.
If two vertices of a parallelogram are (3, 2) (-1, 0) and the diagonals cut at (2, -5), find the other vertices of the parallelogram.
In the given figure, line APB meets the x-axis at point A and y-axis at point B. P is the point (−4, 2) and AP : PB = 1 : 2. Find the co-ordinates of A and B.
A line segment joining A`(-1,5/3)` and B(a, 5) is divided in the ratio 1 : 3 at P, the point where the line segment AB intersects the y-axis.
- Calculate the value of ‘a’.
- Calculate the co-ordinates of ‘P’.
Find the ratio in which the y-axis divides the line segment joining the points (−4, − 6) and (10, 12). Also find the coordinates of the point of division ?
The three vertices of a parallelogram ABCD are A(3, −4), B(−1, −3) and C(−6, 2). Find the coordinates of vertex D and find the area of ABCD.
Find the coordinate of a point P which divides the line segment joining :
D(-7, 9) and E( 15, -2) in the ratio 4:7.
The points A, B and C divides the line segment MN in four equal parts. The coordinates of Mand N are (-1, 10) and (7, -2) respectively. Find the coordinates of A, B and C.
The points A(x1, y1), B(x2, y2) and C(x3, y3) are the vertices of ∆ABC. What are the coordinates of the centroid of the triangle ABC?
