Advertisements
Advertisements
प्रश्न
Show that the line segment joining the points (-3, 10) and (6, -5) is trisected by the coordinates axis.
उत्तर
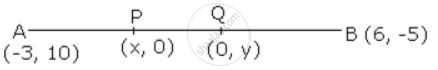
Let the coordinates of two points x-axis and y-axis be P (x, O) and G (0, y) respectively.
Let P divides AB in the ratio k : 1.
Coordinates of P are
P (x , 0) = P `((6"k" - 3)/("k" + 1) , (- 5 "k" + 10)/("k" + 1))`
⇒ 0 = `(- 5 "k" + 10)/("k" + 1)`
⇒ 5 k = 10
⇒ k = 2
Hence P divides AB in the ratio 2: 1.
Let Q divides AB in the ratio k1 : 1.
Coordinates of Q are,
Q (0 , y) = Q `((6"k"_1 - 3)/("k" + 1) , (-5"k" + 10)/("k" + 1))`
`=> 0 = (6"k"_1 - 3)/("k" + 1)`
⇒ 6k1 = 3
⇒ `"k"_1 = 1/2`
Hence Q divides AB in the ratio 1: 2
Hence proved, P and Q are the points of trisection.
APPEARS IN
संबंधित प्रश्न
Find the coordinates of the point which divides the line segment joining the points (6, 3) and (– 4, 5) in the ratio 3 : 2 internally.
Calculate the ratio in which the line joining A(6, 5) and B(4, –3) is divided by the line y = 2.
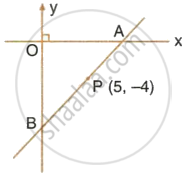
The point P (5, – 4) divides the line segment AB, as shown in the figure, in the ratio 2 : 5. Find the co-ordinates of points A and B. Given AP is smaller than BP.
Find the coordinate of a point P which divides the line segment joining :
A(-8, -5) and B (7, 10) in the ratio 2:3.
The origin o (0, O), P (-6, 9) and Q (12, -3) are vertices of triangle OPQ. Point M divides OP in the ratio 1: 2 and point N divides OQ in the ratio 1: 2. Find the coordinates of points M and N. Also, show that 3MN = PQ.
Find the points of trisection of the segment joining A ( -3, 7) and B (3, -2).
Find the ratio in which the line segment joining P ( 4, -6) and Q ( -3, 8) is divided by the line y = 0.
In what ratio is the line joining (2, -1) and (-5, 6) divided by the y axis ?
If the points A(1, 2), O(0, 0), C(a, b) are collinear, then ______.
What is the ratio in which the line segment joining (2, -3) and (5, 6) is divided by x-axis?
