Advertisements
Advertisements
प्रश्न
Find the coordinate of a point P which divides the line segment joining :
M( -4, -5) and N (3, 2) in the ratio 2 : 5.
उत्तर
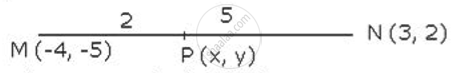
Let the point P divides the line segment MN in the ratio2: 5.
cooordinates of P are
x = `(2 xx 3 + 5 xx -4)/(2 + 5) = -14 / 7 = -2`
y = `(2 xx 2 + 5 xx -5)/(2 + 5) = -3`
APPEARS IN
संबंधित प्रश्न
Find the coordinates of a point P on the line segment joining A(1, 2) and B(6, 7) such that AP =(2/5)AB.
The three vertices of a parallelogram taken in order are (–1, 0), (3, 1) and (2, 2) respectively. Find the coordinates of the fourth vertex.
Find the lengths of the medians of a triangle whose vertices are A (−1,3), B(1,−1) and C(5, 1).
Points A, B, C and D divide the line segment joining the point (5, –10) and the origin in five equal parts. Find the co-ordinates of B and D.
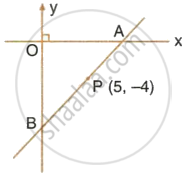
The point P (5, – 4) divides the line segment AB, as shown in the figure, in the ratio 2 : 5. Find the co-ordinates of points A and B. Given AP is smaller than BP.
Find the co-ordinates of the points of tri-section of the line joining the points (–3, 0) and (6, 6).
In what ratio is the line joining (2, -4) and (-3, 6) divided by the line y = O ?
A (30, 20) and B ( 6, -4) are two fixed points. Find the coordinates of a point Pin AB such that 2PB = AP. Also, find the coordinates of some other point Qin AB such that AB = 6 AQ.
The points A(x1, y1), B(x2, y2) and C(x3, y3) are the vertices of ∆ABC. The median from A meets BC at D. Find the coordinates of the point D.
The points A(x1, y1), B(x2, y2) and C(x3, y3) are the vertices of ∆ABC. Find the coordinates of points Q and R on medians BE and CF, respectively such that BQ : QE = 2 : 1 and CR : RF = 2 : 1
