Advertisements
Advertisements
Question
Find the coordinate of a point P which divides the line segment joining :
M( -4, -5) and N (3, 2) in the ratio 2 : 5.
Solution
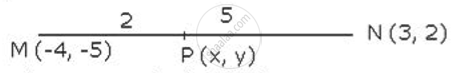
Let the point P divides the line segment MN in the ratio2: 5.
cooordinates of P are
x = `(2 xx 3 + 5 xx -4)/(2 + 5) = -14 / 7 = -2`
y = `(2 xx 2 + 5 xx -5)/(2 + 5) = -3`
APPEARS IN
RELATED QUESTIONS
Find the coordinates of the point which divides the line segment joining the points (6, 3) and (– 4, 5) in the ratio 3 : 2 internally.
Prove that the points (–2, –1), (1, 0), (4, 3) and (1, 2) are the vertices of a parallelogram. Is it a rectangle ?
A point P divides the line segment joining the points A(3, -5) and B(-4, 8) such that `(AP)/(PB) = k/1`. If P lies on the line x + y = 0, then find the value of k.
Find the co-ordinates of the points of tri-section of the line joining the points (–3, 0) and (6, 6).
If A = (−4, 3) and B = (8, −6)
- Find the length of AB.
- In what ratio is the line joining A and B, divided by the x-axis?
Find the ratio in which the line segment joining P ( 4, -6) and Q ( -3, 8) is divided by the line y = 0.
Using section formula, show that the points A(7, −5), B(9, −3) and C(13, 1) are collinear
The points (-5, 1), (1, p) and (4, -2) are collinear if the value of p is ______.
Point C divides the line segment whose points are A(4, –6) and B(5, 9) in the ratio 2:1. Find the coordinates of C.
What is the ratio in which the line segment joining (2, -3) and (5, 6) is divided by x-axis?
