Advertisements
Advertisements
Question
Find the ratio in which the line segment joining P ( 4, -6) and Q ( -3, 8) is divided by the line y = 0.
Solution
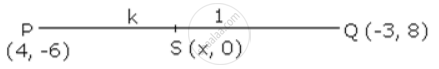
Given PQ is divided by the line Y = O i.e. x-axis.
Let S (x, O) be the pcint on line Y = 0, which divides the line segment PQ in the ratio k : 1.
Coordinates of S are
x = `(-3"k" + 4)/("k" + 1) , 0 = (8"k" - 6)/("k" + 1)`
⇒ 8 k = 6
⇒ k = `3/4`
Hence, the required ratio is 3: 4.
APPEARS IN
RELATED QUESTIONS
In what ratio does the point `(24/11, y)` divide the line segment joining the points P(2, –2) and Q(3, 7)? Also find the value of y.
The line segment joining the points M(5, 7) and N(–3, 2) is intersected by the y-axis at point L. Write down the abscissa of L. Hence, find the ratio in which L divides MN. Also, find the co-ordinates of L.
A line segment joining A`(-1,5/3)` and B(a, 5) is divided in the ratio 1 : 3 at P, the point where the line segment AB intersects the y-axis.
- Calculate the value of ‘a’.
- Calculate the co-ordinates of ‘P’.
In what ratio does the point (1, a) divided the join of (−1, 4) and (4, −1) Also, find the value of a.
The points A, B and C divides the line segment MN in four equal parts. The coordinates of Mand N are (-1, 10) and (7, -2) respectively. Find the coordinates of A, B and C.
Find the ratio in which Y-axis divides the point A(3, 5) and point B(– 6, 7). Find the coordinates of the point
The point which divides the line segment joining the points (7, –6) and (3, 4) in ratio 1 : 2 internally lies in the ______.
If the points A(1, –2), B(2, 3) C(a, 2) and D(– 4, –3) form a parallelogram, find the value of a and height of the parallelogram taking AB as base.
Point C divides the line segment whose points are A(4, –6) and B(5, 9) in the ratio 2:1. Find the coordinates of C.
What is the ratio in which the line segment joining (2, -3) and (5, 6) is divided by x-axis?
