Advertisements
Advertisements
Question
Find the coordinate of a point P which divides the line segment joining :
A (3, -3) and B (6, 9) in the ratio 1 :2.
Solution
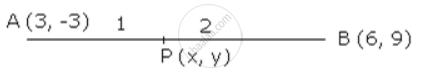
Let the point P divides the line segment AB in the ratio 1: 2.
∴ coordinates of Pare
x = `(1 xx 6 + 2 xx 3)/(1 + 2) = 4`
y = `(1 xx 9 + 2"x" - 3)/(1 + 2) = 1`
APPEARS IN
RELATED QUESTIONS
To conduct Sports Day activities, in your rectangular shaped school ground ABCD, lines have been drawn with chalk powder at a distance of 1 m each. 100 flower pots have been placed at a distance of 1 m from each other along AD, as shown in the following figure. Niharika runs `1/4` th the distance AD on the 2nd line and posts a green flag. Preet runs `1/5` th the distance AD on the eighth line and posts a red flag. What is the distance between both the flags? If Rashmi has to post a blue flag exactly halfway between the line segment joining the two flags, where should she post her flag?

Find the ratio in which the line segment joining the points (-3, 10) and (6, -8) is divided by (-1, 6).
In what ratio is the join of (4, 3) and (2, –6) divided by the x-axis? Also, find the co-ordinates of the point of intersection.
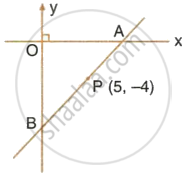
The point P (5, – 4) divides the line segment AB, as shown in the figure, in the ratio 2 : 5. Find the co-ordinates of points A and B. Given AP is smaller than BP.
Find the co-ordinates of the points of tri-section of the line joining the points (–3, 0) and (6, 6).
The line joining P(–4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendicular from P and Q on the x-axis Find:
- the ratio PR : RQ
- the coordinates of R.
- the area of the quadrilateral PMNQ.
If the coordinates of one end of a diameter of a circle are (2, 3) and the coordinates of its centre are (−2, 5), then the coordinates of the other end of the diameter are:
In the figure given below, the line segment AB meets X-axis at A and Y-axis at B. The point P (- 3, 4) on AB divides it in the ratio 2 : 3. Find the coordinates of A and B.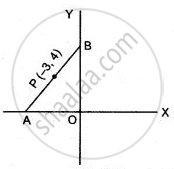
If the points A(1, –2), B(2, 3) C(a, 2) and D(– 4, –3) form a parallelogram, find the value of a and height of the parallelogram taking AB as base.
Find the ratio in which the line segment joining the points A(6, 3) and B(–2, –5) is divided by x-axis.
