Advertisements
Advertisements
प्रश्न
Find the equation of a line passing through (8,3) and making an angle of 45° with the positive direction of the y-axis.
उत्तर
m = tan 45° = 1
Equation of line, `("y" - "y"_1)/("x" - "x"_1)` = m
`("y" - 3)/("x" - 8) = 1`
⇒ x - 8 = y - 3
⇒ x - y = 5
APPEARS IN
संबंधित प्रश्न
The line segment joining the points (5, −4) and (2, 2) is divided by the points Q in the ratio 1 : 2. Does the line x – 2y = 0 contain Q?
Find the equation of the perpendicular dropped from the point (−1, 2) onto the line joining the points (1, 4) and (2, 3).
Find the equation of the line whose slope is `-5/6` and x-intercept is 6.
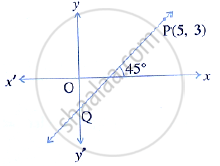
The line through P (5, 3) intersects y-axis at Q.
Find the co-ordinates of Q.
The line 5x - 3y +1 = 0 divides the join of (2,m) and (7,9) in the ratio 2: 3. Find the value of m.
Find the inclination of a line whose gradient is 5.6713
Find the value of a line parallel to the following line:
x = `"y"/2` - 5
Find the value of a line parallel to the following line:
`(3"y")/4 + (5"y")/2 = 7`
Find the equation of a straight line which cuts an intercept of 5 units on Y-axis and is parallel to the line joining the points (3, – 2) and (1, 4).
Find the equation of a line passing through (3, – 2) and perpendicular to the line.
x - 3y + 5 = 0.
