Advertisements
Advertisements
प्रश्न
Find the value of k for which the lines kx – 5y + 4 = 0 and 5x – 2y + 5 = 0 are perpendicular to each other.
उत्तर
kx − 5y + 4 = 0
`=>` 5y = kx + 4
`=> y = k/5 x + 4/5`
Slope of this line = `m_1 = k/5`
5x − 2y + 5 = 0
`=>` 2y = 5x + 5
`=> y = 5/2 x + 5/2`
Slope of this line = `m_2 = 5/2`
Since, the lines are perpendicular, m1 × m2 = –1
`=> k/5 xx 5/2 = -1`
`=>` k = –2
APPEARS IN
संबंधित प्रश्न
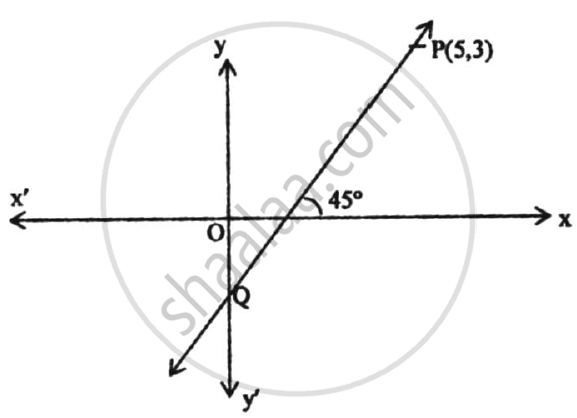
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
The line passing through (0, 2) and (−3, −1) is parallel to the line passing through (−1, 5) and (4, a). Find a.
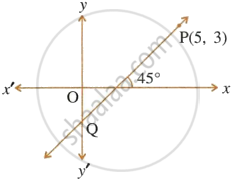
The line through P(5, 3) intersects y-axis at Q.
- Write the slope of the line.
- Write the equation of the line.
- Find the co-ordinates of Q.
Find the slope of the lines passing through the given point.
C (5, –2) , D (7, 3)
Show that the line joining the points A(4, 8) and B(5, 5) is parallel to the line joining the points C(2, 4) and D(1, 7).
A line passing through the points (a, 2a) and (- 2, 3) is perpendicular to the line 4a + 3y + 5 = 0. Find the value of a.
The line through P (5, 3) intersects Y axis at Q.
(i) Write the slope of the line.
(ii) Write the equation of the line.
(iii) Find the coordinates of Q.
Find the slope of the line passing through given points G(3, 7) and K(–2, –3).
Determine whether the following points are collinear. A(–1, –1), B(0, 1), C(1, 3)
Given: Points A(–1, –1), B(0, 1) and C(1, 3)
Slope of line AB = `(square - square)/(square - square) = square/square` = 2
Slope of line BC = `(square - square)/(square - square) = square/square` = 2
Slope of line AB = Slope of line BC and B is the common point.
∴ Points A, B and C are collinear.
