Advertisements
Advertisements
प्रश्न
Given a uniform electric field `vecE=5xx10^3hati`N/C, find the flux of this field through a square of 10 cm on a side whose plane is parallel to the y-z plane. What would be the flux through the same square if the plane makes a 30° angle with the x-axis ?
उत्तर
When the plane is parallel to the y-z plane:
Electric flux, `phi=vecE.vecA`
Here:
`vecE=5xx10^3hati "N/C"`
`vecA=(10cm^2)hati=10^(-2)hatim^2`
`:.phi=(5xx10^3hati).(10^(-2)hati)`
⇒ ϕ =50 Weber
When the plane makes a 30° angle with the x-axis, the area vector makes 60° with the x-axis.
`phi=vecE.vecA`
⇒ϕ=EA cosθ
⇒ϕ=(5×103)(10−2)cos60°
`=>phi=50/2`
⇒ ϕ =25 Weber
APPEARS IN
संबंधित प्रश्न
Define electric flux.
What is the electric flux through a cube of side 1 cm which encloses an electric dipole?
Given the electric field in the region `vecE=2xhati`, find the net electric flux through the cube and the charge enclosed by it.
Two charges of magnitudes −3Q and + 2Q are located at points (a, 0) and (4a, 0) respectively. What is the electric flux due to these charges through a sphere of radius ‘5a’ with its centre at the origin?
A small plane area is rotated in an electric field. In which orientation of the area, is the flux of the electric field through the area maximum? In which orientation is it zero?
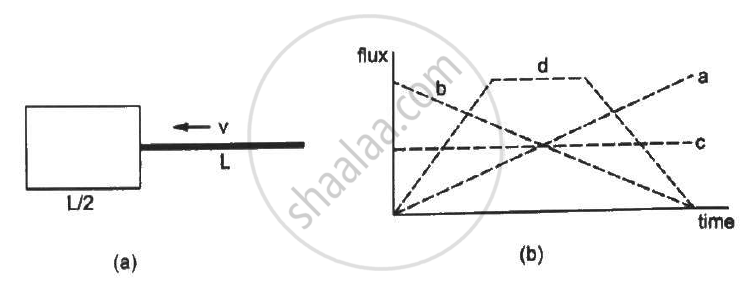
Following Figure (a) shows an imaginary cube of edge L/2. A uniformly charged rod of length (L) moves towards the left at a small but constant speed `nu.` At t = 0, the left end just touches the centre of the face of the cube opposite it. Which of the graphs shown in the figure (b) represents the flux of the electric field through the cube as the rod goes through it?
If the flux of the electric field through a closed surface is zero,
(a) the electric field must be zero everywhere on the surface
(b) the electric field may be zero everywhere on the surface
(c) the charge inside the surface must be zero
(d) the charge in the vicinity of the surface must be zero
The electric field intensity due to an infinite cylinder of radius R and having charge q per unit length at a distance rir r(r > R) from its axis is ______.
What will be the total flux through the faces of the cube (figure) with side of length a if a charge q is placed at

- A: a corner of the cube.
- B: mid-point of an edge of the cube.
- C: centre of a face of the cube.
- D: mid-point of B and C.
A hollow sphere of radius R has a point charge q at its centre. Electric flux emanating from the sphere is X. How will the electric flux change, if at all, when charge q is replaced by an electric dipole?
