Advertisements
Advertisements
प्रश्न
If radii of two cylinders are in the ratio 4 : 3 and their heights are in the ratio 5: 6, find the ratio of their curved surfaces.
उत्तर
The ratio in radii of two cylinders = 4 : 3
and ratio in their heights = 5: 6
Let r1 and r2 be the radii and h1,h2 be their heights respectively.
∴ r1 : r2 = 4:3 and h1 : h2 = 5:6
∴ `r_1 = 4/3 "and" (h_1)/(h_2) = 5/6`
∴ Surface area of the first cylinder = `2pir_1h_1`
and area of second cylinder = `2pir_2h_2`
`(2pir_1h_1)/(2pir_2h_2) = r_1/r_2 xx h_1/h_2 = 4/3 xx 5/6 = 20/18`
= `10/9 = 10 : 9`
∴ Ratio in their surface areas = 10 : 9
APPEARS IN
संबंधित प्रश्न
The paint in a certain container is sufficient to paint an area equal to 9.375 m2. How many bricks of dimensions 22.5 cm × 10 cm × 7.5 cm can be painted out of this container?
A small indoor greenhouse (herbarium) is made entirely of glass panes (including base) held together with tape. It is 30 cm long, 25 cm wide and 25 cm high. What is the area of the glass?
Parveen wanted to make a temporary shelter for her car, by making a box-like structure with tarpaulin that covers all the four sides and the top of the car (with the front face as a flap which can be rolled up). Assuming that the stitching margins are very small, and therefore negligible, how much tarpaulin would be required to make the shelter of height 2.5 m, with base dimensions 4 m × 3 m?
Three equal cubes are placed adjacently in a row. Find the ratio of total surface area of the new cuboid to that of the sum of the surface areas of the three cubes.
The length and breadth of a hall are in the ratio 4: 3 and its height is 5.5 metres. The cost of decorating its walls (including doors and windows) at Rs. 6.60 per square metre is Rs. 5082. Find the length and breadth of the room.
The paint in a certain container is sufficient to paint on area equal to 9.375 m2. How manybricks of dimension 22.5 cm × 10 cm × 7.5 cm can be painted out of this container?
Find the number of cuboidal boxes measuring 2 cm by 3 cm by 10 cm which can be stored in a carton whose dimensions are 40 cm, 36 cm and 24 cm.
A cuboidal block of solid iron has dimensions 50 cm, 45 cm and 34 cm. How many cuboids of size 5 cm by 3 cm by 2 cm can be obtained from this block? Assume cutting causes no wastage.
The weight of a metal block of size 5 cm by 4 cm by 3 cm is 1 kg. Find the weight of a block of the same metal of size 15 cm by 8 cm by 3 cm.
A village, having a population of 4000, requires 150 litres water per head per day. It has a tank which is 20 m long, 15 m broad and 6 m high. For how many days will the water of this tank last?
An 8 m long cuboidal beam of wood when sliced produces four thousand 1 cm cubes and there is no wastage of wood in this process. If one edge of the beam is 0.5 m, find the third edge.
A cuboidal box is 5 cm by 5 cm by 4 cm. Find its surface area.
The dimensions of a cuboid are in the ratio 5 : 3 : 1 and its total surface area is 414 m2. Find the dimensions.
Show that the product of the areas of the floor and two adjacent walls of a cuboid is the square of its volume.
A tank open at the top is made of iron sheet 4 m wide. If the dimensions of the tank are 12 m × 8 m × 6 m, find the cost of iron sheet at Rs 17.50 per metre.
Find the edge of a cube whose surface area is 432 m2.
If the perimeter of each face of a cube is 32 cm, find its lateral surface area. Note that four faces which meet the base of a cube are called its lateral faces.
The length, width and height of a rectangular solid are in the ratio of 3 : 2 : 1. If the volume of the box is 48cm3, the total surface area of the box is
The dimensions of a Cinema Hall are 100 m, 60 m, and 15 m. How many persons can sit in the hall if each requires 150 m3 of air?
Find the length of each edge of a cube, if its volume is :
(i) 216 cm3
(ii) 1.728 m3
A closed box is cuboid in shape with length = 40 cm, breadth = 30 cm and height = 50 cm. It is made of a thin metal sheet. Find the cost of metal sheet required to make 20 such boxes, if 1 m2 of metal sheet costs Rs. 45.
The dining-hall of a hotel is 75 m long; 60 m broad and 16 m high. It has five – doors 4 m by 3 m each and four windows 3 m by 1.6 m each. Find the cost of :
(i) papering its walls at the rate of Rs.12 per m2;
(ii) carpetting its floor at the rate of Rs.25 per m2.
Find the capacity of a cylindrical container with an internal diameter of 28 cm and a height of 20 cm.
The curved surface area and the volume of a toy, cylindrical in shape, are 132 cm2 and 462 cm3 respectively. Find, its diameter and its length.
Find the volume of a cuboid whose diagonal is `3sqrt(29)"cm"` when its length, breadth and height are in the ratio 2 : 3 : 4.
All six faces of a cuboid are ______ in shape and of ______ area.
Find the capacity of water tank, in litres, whose dimensions are 4.2 m, 3 m and 1.8 m?
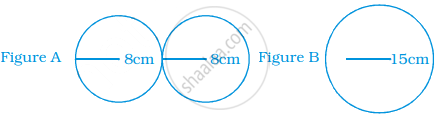
Below are the drawings of cross sections of two different pipes used to fill swimming pools. Figure A is a combination of 2 pipes each having a radius of 8 cm. Figure B is a pipe having a radius of 15 cm. If the force of the flow of water coming out of the pipes is the same in both the cases, which will fill the swimming pool faster?