Advertisements
Advertisements
प्रश्न
If the height of a tower and the distance of the point of observation from its foot, both, are increased by 10%, then the angle of elevation of its top remains unchanged.
पर्याय
True
False
उत्तर
This statement is True.
Explanation:
Case (i): Let the height of a tower be h and the distance of the point of observation from its foot is x.
In ∆ABC,
tan θ1 = `"AC"/"BC" = "h"/x` ...(i)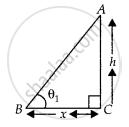
Case (ii): Now, the height of a tower increased by 10%
= h + 10% of h
= `"h" + "h" * 10/100`
= `(11"h")/100`
And the distance of the point of observation from its foot
= x + 10% of x
= `x + x xx 10/100 = (11x)/10`
In ΔPQR,
tan θ2 = `"PR"/"QR" = (((11"h")/10))/(((11x)/10))`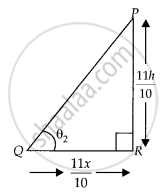
⇒ tan θ2 = `"h"/x` ...(ii)
From equations (i) and (ii), we get
tan θ1 = tan θ2
⇒ θ1 = θ2
Hence, the required angle of elevation of its top remains unchanged.
APPEARS IN
संबंधित प्रश्न
From the top of a building 30 m high, the top and bottom of a tower are observed to have angles of depression 30º and 45º respectively. Find the height of the tower
A man on a cliff observes a fishing trawler at an angle of depression of 30º which is approaching the shore to the point immediately beneath the observer with a uniform speed. 6 minutes later, the angle of depression of the trawler is found to be 60º. Caliculate the time taken by the trawler to reach the shore
A 1.5 m tall boy is standing at some distance from a 30 m tall building. The angle of elevation from his eyes to the top of the building increases from 30° to 60° as he walks towards the building. Find the distance he walked towards the building.
An observed from the top of a 150 m tall lighthouse, the angles of depression of two ships approaching it are 30° and 45°. If one ship is directly behind the other, find the distance between the two ships.
A tower stands vertically on the ground. From a point on the ground which is 20 m away from the foot of the tower, the angle of elevation of its top is found to be 60°. Find the height of the tower. [Take `sqrt(3)` =1.732 ]
Two poles of equal heights are standing opposite to each other on either side of the road which is 80m wide, From a point P between them on the road, the angle of elevation of the top of one pole is 60 and the angle of depression from the top of another pole at P is 30 . Find the height of each pole and distance of the point P from the poles.
A solid right-circular cone of height 60 cm and radius 30 cm is dropped in a right-circular cylinder full of water of height 180 cm and radius 60 cm. Find the volume of water left in the cylinder, in cubic metres ?
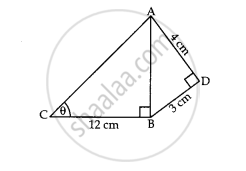
In given figure, AD = 4 cm, BD = 3 cm and CB = 12 cm. The value of tan `theta` is ____________.
If a man standing on a platform 3 meters above the surface of a lake observes a cloud and its reflection in the lake, then calculate the angle of elevation of the cloud.
A vertical tower stands on a horizontal plane and is surmounted by a vertical flagstaff of height h. At a point on the plane, the angles of elevation of the bottom and the top of the flagstaff are α and β, respectively. Then the height of the tower is ____________.
