Advertisements
Advertisements
प्रश्न
If the perpendicular distance of a point P from the x-axis is 5 units and the foot of the perpendicular lies on the negative direction of x-axis, then the point P has ______.
पर्याय
x-coordinate = –5
y-coordinate = 5 only
y-coordinate = –5 only
y-coordinate = 5 or –5
उत्तर
If the perpendicular distance of a point P from the x-axis is 5 units and the foot of the perpendicular lies on the negative direction of x-axis, then the point P has y-coordinate = 5 or –5.
Explanation:
We know that, the perpendicular distance of a point from the x-axis gives y-coordinate of that point.
Here, foot of perpendicular lies on the negative direction of x-axis, so perpendicular distance can be measure in II quadrant or III quadrant.
Hence, the point P has y-coordinate = 5 or –5.
APPEARS IN
संबंधित प्रश्न
On which axis do the following points lie?
P(5, 0)
Find the value of k, if the point P (0, 2) is equidistant from (3, k) and (k, 5).
In what ratio is the line segment joining A(2, -3) and B(5, 6) divide by the x-axis? Also, find the coordinates of the pint of division.
Find the value(s) of k for which the points (3k − 1, k − 2), (k, k − 7) and (k − 1, −k − 2) are collinear.
If the points P (x, y) is equidistant from A (5, 1) and B (−1, 5), then
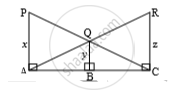
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
Point (–10, 0) lies ______.
Ordinate of all points on the x-axis is ______.
Statement A (Assertion): If the coordinates of the mid-points of the sides AB and AC of ∆ABC are D(3, 5) and E(–3, –3) respectively, then BC = 20 units.
Statement R (Reason): The line joining the mid-points of two sides of a triangle is parallel to the third side and equal to half of it.
Distance of the point (6, 5) from the y-axis is ______.
