Advertisements
Advertisements
प्रश्न
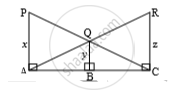
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
उत्तर
In ΔPAC and ΔQBC
∠PCA = ∠QCB
∠PAC = ∠QBC
ΔPAC congurent to ΔQBC
`(PA)/(QB) = (AC)/(BC)`
`x/y = (AB)/(BC)`
`y/x = (BC)/(AC)`
In ΔRCA and ΔQBA
∠RAC = ∠QAB
∠RCA = ∠QBA
ΔRCA is congruent to ΔQBA
`(RC)/(QB) = (AC)/(AB)`
`z/y= (AC)/(AB)`
`y/z= (AB)/(AC)`
adding both eq
`y/z + y/z = (BC + AC)/(AC) = 1`
`y/z + y/z = 1`
multiplying both sides by y
`1/x + 1/z = 1/y`
APPEARS IN
संबंधित प्रश्न
Find the equation of the perpendicular bisector of the line segment joining points (7, 1) and (3,5).
Prove that the points A(-4,-1), B(-2, 4), C(4, 0) and D(2, 3) are the vertices of a rectangle.
If three consecutive vertices of a parallelogram are (1, -2), (3, 6) and (5, 10), find its fourth vertex.
If the poin A(0,2) is equidistant form the points B (3, p) and C (p ,5) find the value of p. Also, find the length of AB.
Show that the points A(6,1), B(8,2), C(9,4) and D(7,3) are the vertices of a rhombus. Find its area.
The midpoint of the line segment joining A (2a, 4) and B (-2, 3b) is C (1, 2a+1). Find the values of a and b.
If the points A(4,3) and B( x,5) lie on the circle with center O(2,3 ) find the value of x .
Find the coordinates of the centre of the circle passing through the points P(6, –6), Q(3, –7) and R (3, 3).
The abscissa of any point on y-axis is
The area of the triangle formed by the points A(2,0) B(6,0) and C(4,6) is
Find the value(s) of k for which the points (3k − 1, k − 2), (k, k − 7) and (k − 1, −k − 2) are collinear.
\[A\left( 6, 1 \right) , B(8, 2) \text{ and } C(9, 4)\] are three vertices of a parallelogram ABCD . If E is the mid-point of DC , find the area of \[∆\] ADE.
If the distance between points (x, 0) and (0, 3) is 5, what are the values of x?
If the centroid of the triangle formed by (7, x) (y, −6) and (9, 10) is at (6, 3), then (x, y) =
If P is a point on x-axis such that its distance from the origin is 3 units, then the coordinates of a point Q on OY such that OP = OQ, are
If the centroid of the triangle formed by the points (3, −5), (−7, 4), (10, −k) is at the point (k −1), then k =
If P(2, 4), Q(0, 3), R(3, 6) and S(5, y) are the vertices of a parallelogram PQRS, then the value of y is
What is the form of co-ordinates of a point on the X-axis?
Write the X-coordinate and Y-coordinate of point P(– 5, 4)
Distance of the point (6, 5) from the y-axis is ______.
