Advertisements
Advertisements
प्रश्न
The area of the triangle formed by the points A(2,0) B(6,0) and C(4,6) is
पर्याय
24 sq. units
12 sq. units
10 sq. units
none of these
उत्तर
Given that points A(2,0), B(6,0) and C(4 ,6) form a triangle which is shown in the figure. We are asked to find the area of the triangle ΔABC.
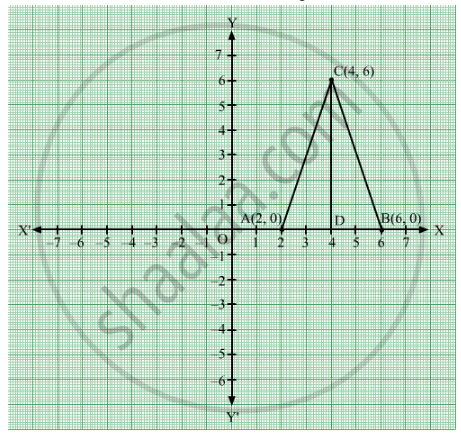
Given that
OA = 2
and OB = 6
Hence
\[\text { AB = OB - OA}\]
\[ = 6 - 2\]
\[ = 4\]
CD = 6
By using formula,
\[∆\text { ABC }= \frac{1}{2} \times \text { AB} \times \text{CD}\]
\[ = \frac{1}{2} \times 4 \times 6\]
\[ = 12 \text { sq units} \]
APPEARS IN
संबंधित प्रश्न
Find the distance between the following pair of points:
(a, 0) and (0, b)
Show that the following points are the vertices of a square:
(i) A (3,2), B(0,5), C(-3,2) and D(0,-1)
Find the area of quadrilateral ABCD whose vertices are A(-5, 7), B(-4, -5) C(-1,-6) and D(4,5)
Points (−4, 0) and (7, 0) lie
If A(3, y) is equidistant from points P(8, −3) and Q(7, 6), find the value of y and find the distance AQ.
If R (x, y) is a point on the line segment joining the points P (a, b) and Q (b, a), then prove that x + y = a + b.
If the points A(−1, −4), B(b, c) and C(5, −1) are collinear and 2b + c = 4, find the values of b and c.
If the centroid of the triangle formed by the points (3, −5), (−7, 4), (10, −k) is at the point (k −1), then k =
(–1, 7) is a point in the II quadrant.
Ryan, from a very young age, was fascinated by the twinkling of stars and the vastness of space. He always dreamt of becoming an astronaut one day. So, he started to sketch his own rocket designs on the graph sheet. One such design is given below :
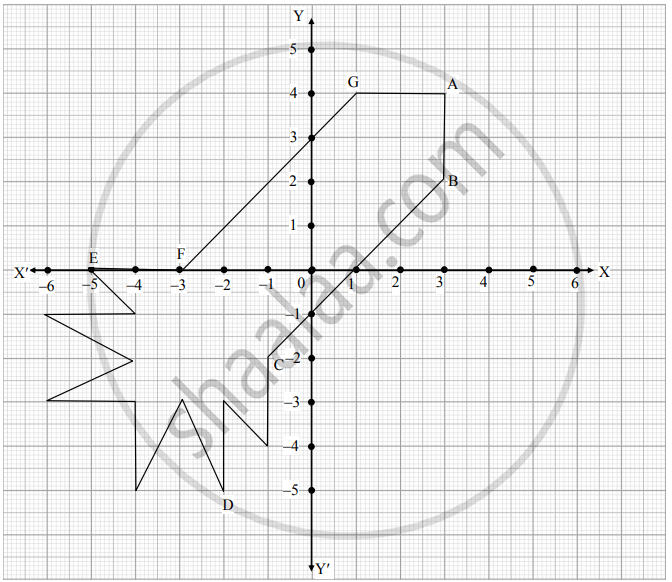
Based on the above, answer the following questions:
i. Find the mid-point of the segment joining F and G. (1)
ii. a. What is the distance between the points A and C? (2)
OR
b. Find the coordinates of the points which divides the line segment joining the points A and B in the ratio 1 : 3 internally. (2)
iii. What are the coordinates of the point D? (1)
