Advertisements
Advertisements
प्रश्न
The area of the triangle formed by the points A(2,0) B(6,0) and C(4,6) is
विकल्प
24 sq. units
12 sq. units
10 sq. units
none of these
उत्तर
Given that points A(2,0), B(6,0) and C(4 ,6) form a triangle which is shown in the figure. We are asked to find the area of the triangle ΔABC.
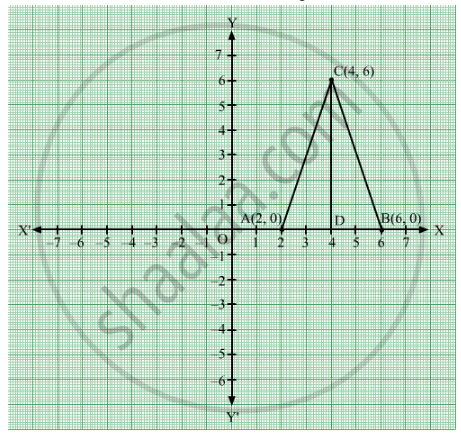
Given that
OA = 2
and OB = 6
Hence
\[\text { AB = OB - OA}\]
\[ = 6 - 2\]
\[ = 4\]
CD = 6
By using formula,
\[∆\text { ABC }= \frac{1}{2} \times \text { AB} \times \text{CD}\]
\[ = \frac{1}{2} \times 4 \times 6\]
\[ = 12 \text { sq units} \]
APPEARS IN
संबंधित प्रश्न
(Street Plan): A city has two main roads which cross each other at the centre of the city. These two roads are along the North-South direction and East-West direction.
All the other streets of the city run parallel to these roads and are 200 m apart. There are 5 streets in each direction. Using 1cm = 200 m, draw a model of the city on your notebook. Represent the roads/streets by single lines.
There are many cross- streets in your model. A particular cross-street is made by two streets, one running in the North - South direction and another in the East - West direction. Each cross street is referred to in the following manner : If the 2nd street running in the North - South direction and 5th in the East - West direction meet at some crossing, then we will call this cross-street (2, 5). Using this convention, find:
- how many cross - streets can be referred to as (4, 3).
- how many cross - streets can be referred to as (3, 4).
Find the ratio in which the line segment joining (-2, -3) and (5, 6) is divided by x-axis Also, find the coordinates of the point of division in each case.
Show that the following points are the vertices of a square:
(i) A (3,2), B(0,5), C(-3,2) and D(0,-1)
Find the co-ordinates of the point which divides the join of A(-5, 11) and B(4,-7) in the ratio 7 : 2
In what ratio is the line segment joining A(2, -3) and B(5, 6) divide by the x-axis? Also, find the coordinates of the pint of division.
Find the value of k, if the points A(7, −2), B (5, 1) and C (3, 2k) are collinear.
Write the coordinates of a point on X-axis which is equidistant from the points (−3, 4) and (2, 5).
If A(x, 2), B(−3, −4) and C(7, −5) are collinear, then the value of x is
A line intersects the y-axis and x-axis at P and Q , respectively. If (2,-5) is the mid-point of PQ, then the coordinates of P and Q are, respectively
If y-coordinate of a point is zero, then this point always lies ______.
