Advertisements
Advertisements
प्रश्न
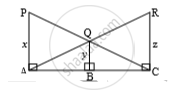
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
उत्तर
In ΔPAC and ΔQBC
∠PCA = ∠QCB
∠PAC = ∠QBC
ΔPAC congurent to ΔQBC
`(PA)/(QB) = (AC)/(BC)`
`x/y = (AB)/(BC)`
`y/x = (BC)/(AC)`
In ΔRCA and ΔQBA
∠RAC = ∠QAB
∠RCA = ∠QBA
ΔRCA is congruent to ΔQBA
`(RC)/(QB) = (AC)/(AB)`
`z/y= (AC)/(AB)`
`y/z= (AB)/(AC)`
adding both eq
`y/z + y/z = (BC + AC)/(AC) = 1`
`y/z + y/z = 1`
multiplying both sides by y
`1/x + 1/z = 1/y`
APPEARS IN
संबंधित प्रश्न
Let ABCD be a square of side 2a. Find the coordinates of the vertices of this square when A coincides with the origin and AB and AD are along OX and OY respectively.
Let ABCD be a square of side 2a. Find the coordinates of the vertices of this square when The centre of the square is at the origin and coordinate axes are parallel to the sides AB and AD respectively.
If two opposite vertices of a square are (5, 4) and (1, −6), find the coordinates of its remaining two vertices.
A (3, 2) and B (−2, 1) are two vertices of a triangle ABC whose centroid G has the coordinates `(5/3,-1/3)`Find the coordinates of the third vertex C of the triangle.
Find the equation of the perpendicular bisector of the line segment joining points (7, 1) and (3,5).
Find the points of trisection of the line segment joining the points:
(3, -2) and (-3, -4)
If A and B are (1, 4) and (5, 2) respectively, find the coordinates of P when AP/BP = 3/4.
Find the points on the x-axis, each of which is at a distance of 10 units from the point A(11, –8).
Find the coordinates of the midpoints of the line segment joining
P(-11,-8) and Q(8,-2)
The base BC of an equilateral triangle ABC lies on y-axis. The coordinates of point C are (0, -3). The origin is the midpoint of the base. Find the coordinates of the points A and B. Also, find the coordinates of another point D such that ABCD is a rhombus.
If (x, y) be on the line joining the two points (1, −3) and (−4, 2) , prove that x + y + 2= 0.
Find the value of k if points A(k, 3), B(6, −2) and C(−3, 4) are collinear.
Write the formula for the area of the triangle having its vertices at (x1, y1), (x2, y2) and (x3, y3).
The coordinates of a point on x-axis which lies on the perpendicular bisector of the line segment joining the points (7, 6) and (−3, 4) are
If the line segment joining the points (3, −4), and (1, 2) is trisected at points P (a, −2) and Q \[\left( \frac{5}{3}, b \right)\] , Then,
If P(2, 4), Q(0, 3), R(3, 6) and S(5, y) are the vertices of a parallelogram PQRS, then the value of y is
Find the coordinates of the point of intersection of the graph of the equation x = 2 and y = – 3
If y-coordinate of a point is zero, then this point always lies ______.
If the vertices of a parallelogram PQRS taken in order are P(3, 4), Q(–2, 3) and R(–3, –2), then the coordinates of its fourth vertex S are ______.
Distance of the point (6, 5) from the y-axis is ______.
