Advertisements
Advertisements
प्रश्न
If the sides of a triangle are produced in an order, show that the sum of the exterior angles so formed is 360°.
उत्तर
In ΔABC, by exterior angle property,
Exterior ∠1 = Interior ∠A + Interior ∠B ...(i)
Exterior ∠2 = Interior ∠B + Interior ∠C ...(ii)
Exterior ∠3 = Interior ∠A + Interior ∠C ...(iii)

On adding equations (i), (ii) and (iii), we get
∠1 + ∠2 + ∠3 = 2(∠A + ∠B + ∠C) ...[By angle sum property of a triangle, ∠A + ∠B + ∠C = 180°]
⇒ ∠1 + ∠2 + ∠3 = 2 × 180°
⇒ ∠1 + ∠2 + ∠3 = 360°
Hence, the sum of exterior angles is 360°.
APPEARS IN
संबंधित प्रश्न
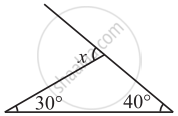
Find the value of the unknown exterior angle x in the following diagram:
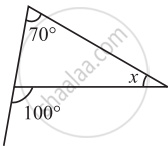
Find the value of the unknown interior angle x in the following figure.
Using the measures of the angles given in the figure alongside, find the measures of the remaining three angles.

Using the given figure find the value of x.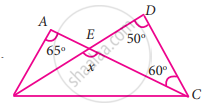
If the exterior angle of a triangle is 140° and its interior opposite angles are equal, find all the interior angles of the triangle
In the figure find the value of x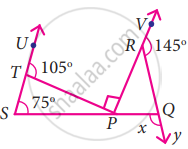
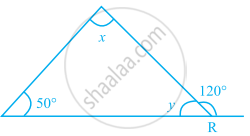
The measures of ∠x and ∠y in the given figure are respectively.
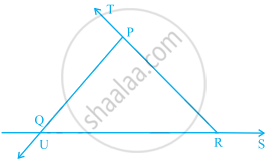
In the given figure, ∠PRS = ∠ ______ + ∠ _______
In ∆ABC, if ∠A = ∠C, and exterior angle ABX = 140°, then find the angles of the triangle.
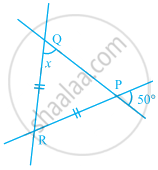
In the given figure, if RP = RQ, find the value of x.