Advertisements
Advertisements
प्रश्न
If the sides of a triangle are produced in an order, show that the sum of the exterior angles so formed is 360°.
उत्तर
In ΔABC, by exterior angle property,
Exterior ∠1 = Interior ∠A + Interior ∠B ...(i)
Exterior ∠2 = Interior ∠B + Interior ∠C ...(ii)
Exterior ∠3 = Interior ∠A + Interior ∠C ...(iii)

On adding equations (i), (ii) and (iii), we get
∠1 + ∠2 + ∠3 = 2(∠A + ∠B + ∠C) ...[By angle sum property of a triangle, ∠A + ∠B + ∠C = 180°]
⇒ ∠1 + ∠2 + ∠3 = 2 × 180°
⇒ ∠1 + ∠2 + ∠3 = 360°
Hence, the sum of exterior angles is 360°.
APPEARS IN
संबंधित प्रश्न
Find the value of the unknown interior angle x in the following figure.
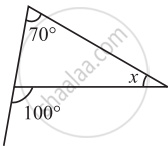
Find the value of the unknown interior angle x in the following figure.

Find the value of the unknown interior angle x in the following figure.

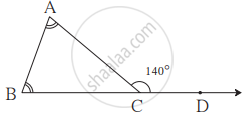
∠ACD is an exterior angle of ∆ABC. The measures of ∠A and ∠B are equal. If m∠ACD = 140°, find the measures of the angles ∠A and ∠B.
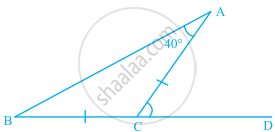
Using the measures of the angles given in the figure alongside, find the measures of the remaining three angles.

An exterior angle of a triangle is 70° and two interior opposite angles are equal. Then measure of each of these angle will be
In the given figure, BC = CA and ∠A = 40°. Then, ∠ACD is equal to ______.
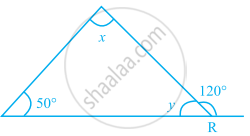
The measures of ∠x and ∠y in the given figure are respectively.
In the given figure, if AB || CD, then ______.
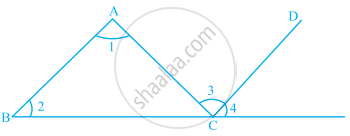
Find the value of x in the given figure.

