Advertisements
Advertisements
प्रश्न
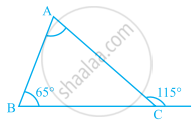
In ∆ABC, if ∠A = ∠C, and exterior angle ABX = 140°, then find the angles of the triangle.
उत्तर
Given, ∠A = ∠C and exterior ∠ABX = 140°
Let ∠A = ∠C = x
According to the exterior angle property,

Exterior ∠B = Interior ∠A + Interior ∠C
⇒ 140° = x + x
⇒ 140° = 2x
⇒ x = `140^circ/2` = 70°
So, ∠A = ∠C = 70°
Now, ∠A + ∠B + ∠C = 180° ......[Angle sum property of a triangle]
⇒ 70 + ∠B + 70° = 180°
⇒ ∠B + 140° = 180°
⇒ ∠B = 180° – 140°
⇒ ∠B = 40°
Hence, all the angles of the triangles are 70°, 40° and 70°.
APPEARS IN
संबंधित प्रश्न
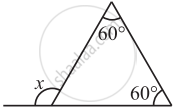
Find the value of the unknown exterior angle x in the following diagram:
In the isosceles triangle ABC, ∠A, and ∠B are equal. ∠ACD is an exterior angle of ∆ABC. The measures of ∠ACB and ∠ACD are (3x − 17)° and (8x + 10)°, respectively. Find the measures of ∠ACB and ∠ACD. Also find the measures of ∠A and ∠B.
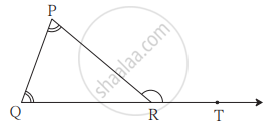
In ∆PQR, the measures of ∠P and ∠Q are equal and m∠PRQ = 70°. Find the measures of the following angles.
- m∠PRT
- m∠P
- m∠Q
Find the value of x in the given triangle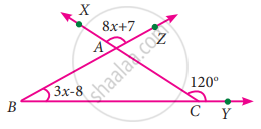
An exterior angle of a triangle is 70° and two interior opposite angles are equal. Then measure of each of these angle will be
In the given figure BD = BC, find the value of x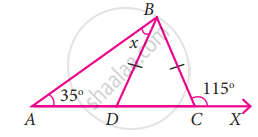
In the given figure find the values of x and y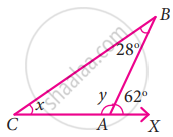
In the given figure, PQ = PR, RS = RQ and ST || QR. If the exterior angle RPU is 140°, then the measure of angle TSR is ______.
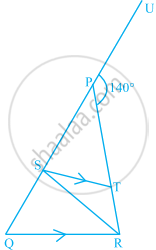
If the exterior angle of a triangle is 130° and its interior opposite angles are equal, then measure of each interior opposite angle is ______.
Find the measure of ∠A in the given figure.