Advertisements
Advertisements
प्रश्न
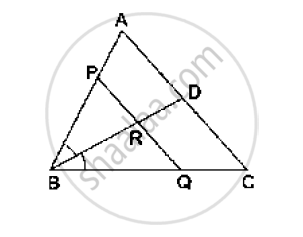
In ΔABC, the bisector of ∠B meets AC at D. A line OQ║AC meets AB, BC and BD at O, Q and R respectively. Show that BP × QR = BQ × PR
उत्तर
In triangle BQO, BR bisects angle B.
Applying angle bisector theorem, we get:
`(QR)/(PR)=(BQ)/(BP)`
⟹BP × QR = BQ × PR
This completes the proof.
shaalaa.com
या प्रश्नात किंवा उत्तरात काही त्रुटी आहे का?
