Advertisements
Advertisements
प्रश्न
In the below Fig, ∠AOC and ∠BOC form a linear pair. if a − 2b = 30°, find a and b.
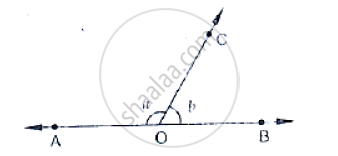
उत्तर
Given that,
`∠`AOC and `∠`BOC form a linear pair
If a - 2b = 30°
`∠`AOC = a°, `∠`BOC = b°
∴a + b = 180° ..........(i)
Given a - 2b = 30° ............(ii)
By subtracting (i) and (ii)
a + b - a + 2b = 180° - 30°
⇒ 3b = 150°
⇒ b = `(150°)/3`
⇒ b = 50°
Hence a - 2b = 30°
a - 2 (50)° = 30° [∴ b = 50°]
a = 30° +100°
a = 130°
∴ a = 130°, b = 50°
APPEARS IN
संबंधित प्रश्न
Write the complement of the following angle.
20°
Write the complement of the following angles .
30°
Write the supplement of the following angles.
132°
An angle is 14° more than its complementary angle. What is its measure?
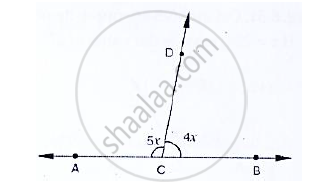
In the below fig, ACB is a line such that ∠DCA = 5x and ∠DCB = 4x. Find the value of x.
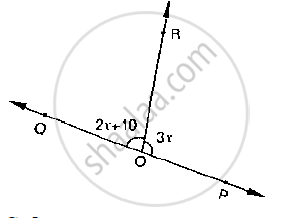
Given ∠POR = 3x and ∠QOR = 2x + 10, find the value of x for which POQ will be a line.
(Below fig).
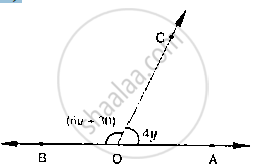
What value of y would make AOB a line in below fig, if ∠AOC = 4y and ∠BOC = (6y +
30)
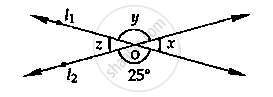
In the given fig, find the values of x, y and z.
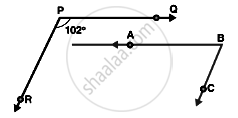
In the below, PQ || AB and PR || BC. If `∠`QPR = 102°, determine `∠`ABC. Give reasons.
The opposite sides of a quadrilateral are parallel. If one angle of the quadrilateral is 60°,
find the other angles.
