Advertisements
Advertisements
Question
In the below Fig, ∠AOC and ∠BOC form a linear pair. if a − 2b = 30°, find a and b.
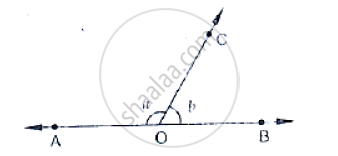
Solution
Given that,
`∠`AOC and `∠`BOC form a linear pair
If a - 2b = 30°
`∠`AOC = a°, `∠`BOC = b°
∴a + b = 180° ..........(i)
Given a - 2b = 30° ............(ii)
By subtracting (i) and (ii)
a + b - a + 2b = 180° - 30°
⇒ 3b = 150°
⇒ b = `(150°)/3`
⇒ b = 50°
Hence a - 2b = 30°
a - 2 (50)° = 30° [∴ b = 50°]
a = 30° +100°
a = 130°
∴ a = 130°, b = 50°
APPEARS IN
RELATED QUESTIONS
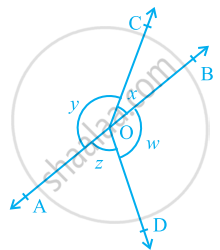
In the given figure, if x + y = w + z, then prove that AOB is a line.
Write the complement of the following angles .
35°
Write the supplement of the following angles .
138°
An angle is 14° more than its complementary angle. What is its measure?
Given ∠POR = 3x and ∠QOR = 2x + 10, find the value of x for which POQ will be a line.
(Below fig).
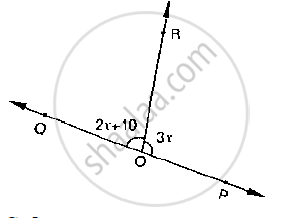
In below fig, ray OS stand on a line POQ. Ray OR and ray OT are angle bisectors of ∠POS
and ∠SOQ respectively. If ∠POS = x, find ∠ROT.

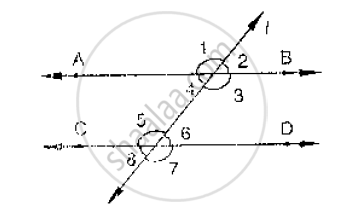
In below fig, AB CD and ∠1 and ∠2 are in the ratio 3 : 2. Determine all angles from 1 to 8.
If below fig, if AB || CD and CD || EF, find ∠ACE.

In the below fig, if l || m, n || p and ∠1 = 85°, find `∠`2.
Fill in the blank in the following to make the statement true:
If two parallel lines are intersected by a transversal, then each pair of corresponding
angles are _______
