Advertisements
Advertisements
Question
An angle is 14° more than its complementary angle. What is its measure?
Solution
Given that,
An angle is 14° more than its complementary angle
The angle measured is ‘x’ say
The complementary angle of ‘x’ is (90 - x)
It is given that
x- (90 - x) = 14
⇒ x - 90 + x = 14
⇒ 2x° = 90° +14°
⇒ x° = `(104°)/2`
⇒ x = 52°.
∴ The angle measured is 52°
APPEARS IN
RELATED QUESTIONS
Write the supplement of the following angles .
54°
If the complement of an angle is equal to the supplement of the thrice of it. Find the measure of the angle.
In the below fig, write all pairs of adjacent angles and all the linear pairs .

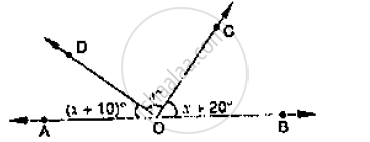
In the given below Fig, find x. Further find ∠BOC, ∠COD and ∠AOD .
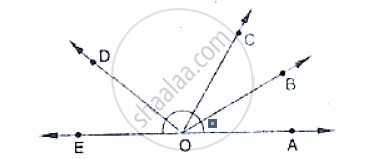
How many pairs of adjacent angles, in all, can you name in below fig.?
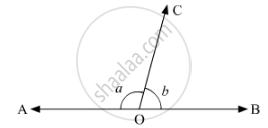
In Fig. 8.42, a is greater than b by one third of a right-angle. Find the values of a and b.
statement are true and false
Angles forming a linear pair can both the acute angles.
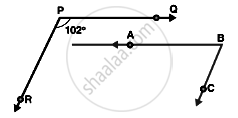
In the below, PQ || AB and PR || BC. If `∠`QPR = 102°, determine `∠`ABC. Give reasons.
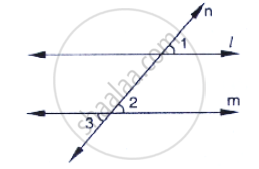
In the below fig, `∠`1 = 60° and `∠`2 = `(2/3)^(rd)`of a right angle. Prove that l || m.
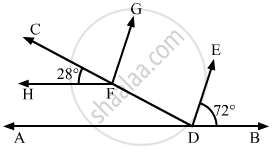
In the given figure, if AB || HF and DE || FG, then the measure of ∠FDE is