Advertisements
Advertisements
प्रश्न
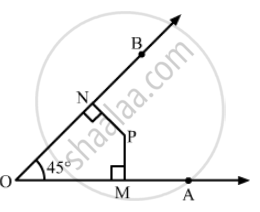
In Fig. 16.20, find the measure of ∠MPN.
उत्तर
\[\text{ Since the sum of all the angles of a quadrilateral is } 360°, \text{ we have } : \]
\[45° + 90° + 90° + ∠MPN = 360° \]
\[ \Rightarrow 225° + ∠MPN = 360°\]
\[ \therefore ∠MPN = 135° \]
APPEARS IN
संबंधित प्रश्न
How many diagonals does following have?
A regular hexagon
ABCD is a parallelogram, AD is produced to E so that DE = DC and EC produced meets AB produced in F. Prove that BF = BC.
Complete of the following, so as to make a true statement:
In a quadrilateral the point of intersection of the diagonals lies in .... of the quadrilateral.
In Fig. 16.19, ABCD is a quadrilateral.
Name a pair of adjacent angles.
Complete the following statement by means of one of those given in brackets against each:
A line drawn from the mid-point of one side of a triangle .............. another side intersects the third side at its mid-point.
Which of the following quadrilateral is not a rhombus?
Write, giving reason, the name of the figure drawn alongside. Under what condition will this figure be a square.
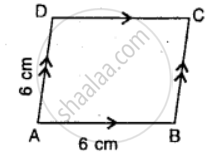
ABCD is a quadrilateral in which AB || DC and AD = BC. Prove that ∠A = ∠B and ∠C = ∠D.
If a bicycle wheel has 48 spokes, then the angle between a pair of two consecutive spokes is ______.
In given figure, What is BD – DE?