Advertisements
Advertisements
प्रश्न
ABCD is a parallelogram, AD is produced to E so that DE = DC and EC produced meets AB produced in F. Prove that BF = BC.
उत्तर
Draw a parallelogram ABCD with AC and BD intersecting at O
Produce AD to E such that DE = DC
Join EC and produce it to meet AB produced at F.
In ΔDCE ,
∴`∠`DCE = `∠`DEC .........CD [In a triangle, equal sides have equal angles opposite]
AB || CD (Opposite sides of the parallelogram are parallel)
∴AE || CD ( AB Lies on AF )
AF || CD and EF is the transversal.
∴`∠`DCE = `∠`BFC .....(2) [Pair of corresponding angles]
From (1) and (2), we get
`∠`DEC = `∠`BFC
In ΔAFE,
`∠`AFE = `∠`AEF (`∠`DEC = `∠`BFC )
∴AE = AF (In a triangle, equal angles have equal sides opposite to them)
⇒ AD + DE = AB + BF
⇒ BC + AB = AB + BF [ ∵ AD = BC, DE = CD and CD = AB, AB = DE ]
⇒ BC = BF
APPEARS IN
संबंधित प्रश्न
In a quadrilateral, define of the following Opposite sides .
Complete of the following, so as to make a true statement:
A quadrilateral is convex if, for each side, the remaining ______ lie on the same side of the line containing the side.
A quadrilateral has three acute angles each measures 80°. What is the measure of the fourth angle?
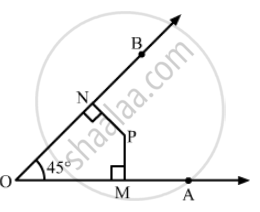
In Fig. 16.20, find the measure of ∠MPN.
The sides of a quadrilateral are produced in order. What is the sum of the four exterior angles?
In Fig. 16.21, the bisectors of ∠A and ∠B meet at a point P. If ∠C = 100° and ∠D = 50°, find the measure of ∠APB.

Two angles of a quadrilateral are 68° and 76°. If the other two angles are in the ratio 5 : 7; find the measure of each of them.
Use the following figure to find the value of x

A diagonal of a rectangle is inclined to one side of the rectangle at 25º. The acute angle between the diagonals is ______.
Draw a rough sketch of a quadrilateral KLMN. State two pairs of opposite sides.
