Advertisements
Advertisements
प्रश्न
In the given figure, AB and DE are perpendicular to BC.

1) Prove that ΔABC ∼ ΔDEC
2) If AB = 6 cm; DE = 4 cm and AC = 15 cm. Calculate CD.
3) Find the ratio of area of ΔABC: area of ΔDEC
उत्तर
1) In ΔABC and ΔDEC,
∠ABC = ∠DEC = 90°`
∠ACB = ∠DCE (Common)
∴ ΔABC ~ ΔDCE (AA criterion)
2) Since ΔABC ∼ ΔDEC,
`"AB"/"DE" = "AC"/"CD"`
`=> 6/4 = 15/"CD" => 6 xx CD = 60 => CD = 60/6 = 10 cm`
3) It is known that the ratio of areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
`=> (Ar(ABC))/(Ar(DEC)) = "AB"^2/"DE"^2 = 6^2/4^2 = 36/16 = 9/4`
`=> ar(ABC) : ar(DEC) = 9 : 4`
APPEARS IN
संबंधित प्रश्न
The diagonal BD of a parallelogram ABCD intersects the segment AE at the point F, where E is any point on the side BC. Prove that DF × EF = FB × FA
Given: FB = FD, AE ⊥ FD and FC ⊥ AD.
Prove that: `(FB)/(AD) = (BC)/(ED)`.

ΔABC is right angled at A and AD⊥BC. If BC = 13cm and AC = 5cm, find the ratio of the areas of ΔABC and ΔADC.
Prove that the area of Δ BCE described on one side BC of a square ABCD is one half the area of the similar Δ ACF described on the diagonal AC.
In the given figure, ΔABC ~ ΔADE. If AE : EC = 4 : 7 and DE = 6.6 cm, find BC. If 'x' be the length of the perpendicular from A to DE, find the length of perpendicular from A to BC in terms of 'x'.

In ΔABC, point D divides AB in the ratio 5:7, Find: DE, If BC = 4.8cm
A model of a ship is made to a scale of 1:500. Find: The volume of the model when the volume of the ship is 1km3
Write the test of similarity for triangles given in figure.
In given fig., quadrilateral PQRS, side PQ || side SR, AR = 5 AP, then prove that, SR = 5PQ
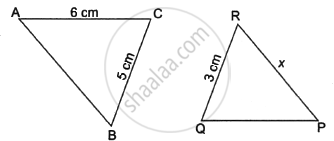
In the given figure, ΔABC ∼ ΔQPR, If AC = 6 cm, BC = 5 cm, QR = 3 cm and PR = x; them the value of x is ______.