Advertisements
Advertisements
प्रश्न
In Δ PQR, bisectors of ∠ PQR and ∠ PRQ meet at I. Prove that I is equidistant from the three sides of the triangle , and PI bisects ∠ QPR .
उत्तर
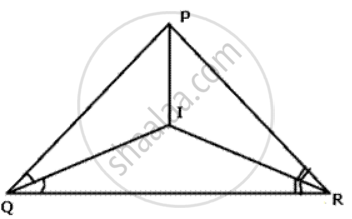
Since I lies on bisector of ∠ R, I is equidistant from PR and QR.
Again I lies on the bisector of ∠ Q , I is equidistant from PQ and QR.
Hence , I is equi distant from alI sides of the triangle.
Therefore, I lies on the bisector of ∠ P i.e ∠ QPR .
APPEARS IN
संबंधित प्रश्न
Describe the locus of a point in space, which is always at a distance of 4 cm from a fixed point.
Describe the locus of a point P, so that:
AB2 = AP2 + BP2,
where A and B are two fixed points.
Draw an angle ABC = 75°. Find a point P such that P is at a distance of 2 cm from AB and 1.5 cm from BC.
Construct a ti.PQR, in which PQ=S. 5 cm, QR=3. 2 cm and PR=4.8 cm. Draw the locus of a point which moves so that it is always 2.5 cm from Q.
In Δ PQR, s is a point on PR such that ∠ PQS = ∠ RQS . Prove thats is equidistant from PQ and QR.
In Δ ABC, B and Care fixed points. Find the locus of point A which moves such that the area of Δ ABC remains the same.
Draw and describe the lorus in the following cases:
The lorus of a point in rhombus ABCD which is equidistant from AB and AD .
State and draw the locus of a swimmer maintaining the same distance from a lighthouse.
State and draw the locus of a point equidistant from two given parallel lines.
Using only a ruler and compass construct ∠ABC = 120°, where AB = BC = 5 cm.
(i) Mark two points D and E which satisfy the condition that they are equidistant from both ABA and BC.
(ii) In the above figure, join AD, DC, AE and EC. Describe the figures:
(a) AECB, (b) ABD, (c) ABE.
