Advertisements
Advertisements
प्रश्न
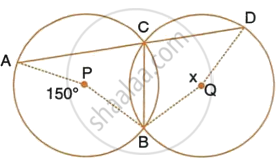
In the figure, given below, P and Q are the centres of two circles intersecting at B and C. ACD is a straight line. Calculate the numerical value of x .
उत्तर
`∠ACB = 1/2 ∠APB = 1/2 xx 150^circ = 75^circ `
(Angle at the centre is double the angle at the circumference subtended by the same chord)
∠ACB + ∠BCD = 180°
(Straight line)
`=>` ∠BCD = 180° – 75° = 105°
Also, ∠BCD = `1/2` reflex ∠BQD = `1/2 (360^circ - x)`
(Angle at the center is double the angle at the circumference subtended by the same chord)
`=> 105^circ = 180^circ - x/2`
∴ x = 2(180° – 105°)
= 2 × 75°
= 150°
APPEARS IN
संबंधित प्रश्न
The figure given below, shows a Circle with centre O.
Given: ∠AOC = a and ∠ABC = b.
Find the relationship between a and b

In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find :
- ∠AOB,
- ∠ACB,
- ∠ABD,
- ∠ADB.
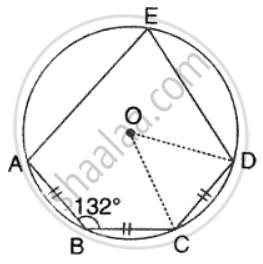
In the given figure, AB = BC = CD and ∠ABC = 132°.
Calcualte:
- ∠AEB,
- ∠AED,
- ∠COD.

The figure shows a circle with centre O. AB is the side of regular pentagon and AC is the side of regular hexagon. Find the angles of triangle ABC.

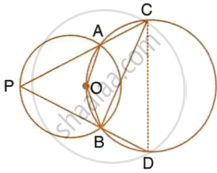
The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given that ∠APB = a°.
Calculate, in terms of a°, the value of : ∠ADB.
Give reasons for your answers clearly.
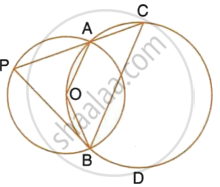
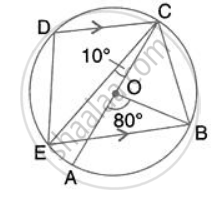
In the given figure, AC is the diameter of circle, centre O. CD and BE are parallel. Angle AOB = 80o and angle ACE = 10o. Calculate : Angle BCD
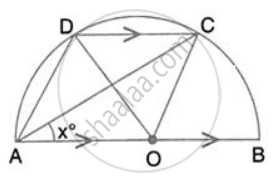
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠ CAB = xo ; find (in terms of x) the values of: ∠ DAC
In the given figure, AB = BC = CD and ∠ABC = 132° . Calcualte: ∠ COD.
C is a point on the minor arc AB of the circle, with centre O. Given ∠ACB = x° and ∠AOB = y° express y in terms of x. Calculate x, if ACBO is a parallelogram.
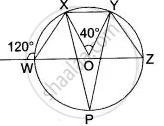
In the figure alongside O is the centre of circle ∠ XOY = 40°, ∠ TWX = 40° and XY is parallel to TZ.
Find: (i) ∠ XZY, (ii) ∠ YXZ (iii) ∠ TZY.