Advertisements
Advertisements
प्रश्न
In the given figure, AB = BC = CD and ∠ABC = 132°.
Calcualte:
- ∠AEB,
- ∠AED,
- ∠COD.

उत्तर
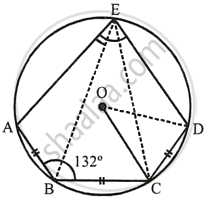
In the figure, O is the centre of circle, with AB = BC = CD.
∠ABC = 132°
i. In cyclic quadrilateral ABCE
∠ABC + ∠AEC = 180° ...[Sum of opposite angles]
`=>` ∠132° + ∠AEC = 180°
`=>` ∠AEC = 180° – 132°
`=>` ∠AEC = 48°
Since, AB = BC, ∠AEB = ∠BEC ...[Equal chords subtends equal angles]
∴ `∠AEB = 1 /2 ∠AEC`
= `1/2 xx 48^circ`
= 24°
ii. Similarly, AB = BC = CD
∠AEB = ∠BEC = ∠CED = 24°
∠AED = ∠AEB + ∠BEC + ∠CED
= 24° + 24° + 24°
= 72°
iii. Arc CD subtends ∠COD at the centre and ∠CED at the remaining part of the circle.
∴ COD = 2∠CED
= 2 × 24°
= 48°
APPEARS IN
संबंधित प्रश्न
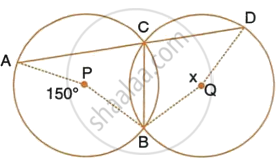
In the figure, given below, P and Q are the centres of two circles intersecting at B and C. ACD is a straight line. Calculate the numerical value of x .
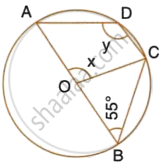
In the given figure, O is the centre of the circle and ∠ABC = 55°. Calculate the values of x and y.
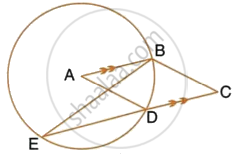
In the given figure, A is the centre of the circle, ABCD is a parallelogram and CDE is a straight line. Prove that : ∠BCD = 2∠ABE.
In the given figure, AE is the diameter of the circle. Write down the numerical value of ∠ABC + ∠CDE. Give reasons for your answer.
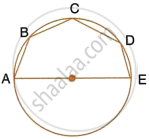
In the given figure, BD is a side of a regular hexagon, DC is a side of a regular pentagon and AD is a diameter.
Calculate :
- ∠ADC,
- ∠BDA,
- ∠ABC,
- ∠AEC.

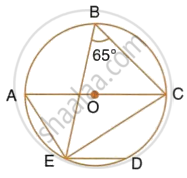
In the given figure, chord ED is parallel to diameter AC of the circle. Given ∠CBE = 65°, calculate ∠DEC.
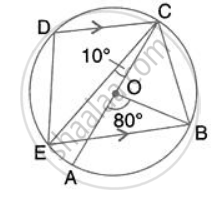
In the given figure, AC is the diameter of circle, centre O. CD and BE are parallel. Angle AOB = 80o and angle ACE = 10o. Calculate : Angle BCD
In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find : ∠ACB

In the given figure, AB = BC = CD and ∠ABC = 132° . Calcualte: ∠AED

Prove that the line segment joining the midpoints of two equal chords of a circle subtends equal angles with the chord.
