Advertisements
Advertisements
प्रश्न
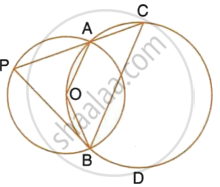
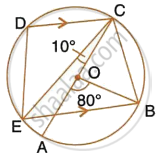
In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find :
- ∠AOB,
- ∠ACB,
- ∠ABD,
- ∠ADB.
उत्तर

Join AB and AD
i. ∠AOB = 2∠APB
= 2 × 75°
= 150°
(Angle at the centre is double the angle at the circumference subtended by the same chord).
ii. In cyclic quadrilateral AOBC,
∠ACB = 180° – ∠AOB
= 180° – 150°
= 30°
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
iii. In cyclic quadrilateral ABDC
∠ABD = 180° – ∠ACD
= 180° – (40° + 30°)
= 110°
(Pair of opposite angles in a cyclic quadrilateral are supplementary
iv. In cyclic quadrilateral AOBD,
∠ADB = 180° – ∠AOB
= 180° – 150°
= 30°
(Pair of opposite angles in a cyclic quadrilateral are supplementary
APPEARS IN
संबंधित प्रश्न
In the figure, O is the centre of the circle, ∠AOE = 150°, ∠DAO = 51°. Calculate the sizes of the angles CEB and OCE.

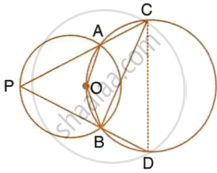
In the figure, given below, P and Q are the centres of two circles intersecting at B and C. ACD is a straight line. Calculate the numerical value of x .
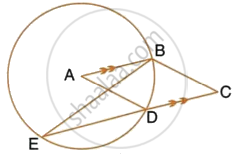
The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given that ∠APB = a°.
Calculate, in terms of a°, the value of : obtuse ∠AOB,
Give reasons for your answers clearly.
In the given figure, A is the centre of the circle, ABCD is a parallelogram and CDE is a straight line. Prove that : ∠BCD = 2∠ABE.
In the given figure, AC is the diameter of the circle with centre O. CD and BE are parallel. Angle ∠AOB = 80° and ∠ACE = 10°.
Calculate:
- Angle BEC,
- Angle BCD,
- Angle CED.
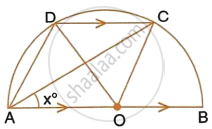
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠CAB = x°; find (in terms of x) the values of :
- ∠COB,
- ∠DOC,
- ∠DAC,
- ∠ADC.
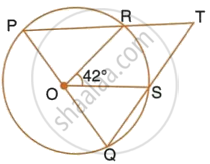
In the given figure, PQ is the diameter of the circle whose centre is O. Given ∠ROS = 42°, calculate ∠RTS.
In the given figure, AB is the diameter of the circle with centre O.
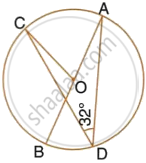
If ∠ADC = 32°, find angle BOC.
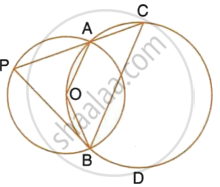
The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given that ∠APB = a°.
Calculate, in terms of a°, the value of : ∠ADB.
Give reasons for your answers clearly.
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠ CAB = xo ; find (in terms of x) the values of: ∠ ADC.