Advertisements
Advertisements
प्रश्न
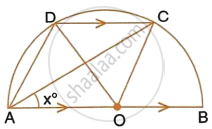
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠CAB = x°; find (in terms of x) the values of :
- ∠COB,
- ∠DOC,
- ∠DAC,
- ∠ADC.
उत्तर

i. ∠COB = 2∠CAB = 2x
(Angle ate the centre is double the angler at the circumference subtended by the same order)
ii. ∠OCD = ∠COB = 2x (Alternate angles)
In ΔOCD, OC = OD
∴ ∠ODC = ∠OCD = 2x
By angle sum property of ∆OCD,
∠DOC = 180° – 2x – 2x = 180° – 4x
iii. `∠DAC = 1/2 ∠DOC`
= `1/2 (180^circ - 4x)`
= 90° – 2x
(Angle at the centre is double the angle at the circumference subtended by the same chord)
iv. DC || AO
∴ ∠ACD = ∠OAC = x (Alternate angles)
By angle sum property,
∠ADC = 180° – ∠DAC – ∠ACD
= 180° – (90° – 2x) – x
= 90° + x
APPEARS IN
संबंधित प्रश्न
In the figure, O is the centre of the circle, ∠AOE = 150°, ∠DAO = 51°. Calculate the sizes of the angles CEB and OCE.

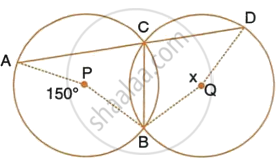
In the figure, given below, P and Q are the centres of two circles intersecting at B and C. ACD is a straight line. Calculate the numerical value of x .
AB is the diameter of the circle with centre O. OD is parallel to BC and ∠AOD = 60°. Calculate the numerical values of :
- ∠ABD,
- ∠DBC,
- ∠ADC.
In the given diagram, chord AB = chord BC.
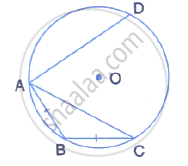
(i) what is the relation between arcs AB and BC?
(ii) what is the relation between ∠AOB and ∠BOC?
(iii) If arc AD is greater than arc ABC, then what is the relation between chords AD and AC?
(iv) If ∠AOB = 50°, find the measure of angle BAC.
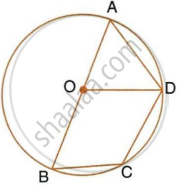
In the given figure, BD is a side of a regular hexagon, DC is a side of a regular pentagon and AD is a diameter.
Calculate :
- ∠ADC,
- ∠BDA,
- ∠ABC,
- ∠AEC.

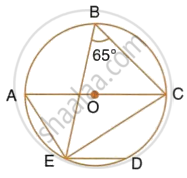
In the given figure, chord ED is parallel to diameter AC of the circle. Given ∠CBE = 65°, calculate ∠DEC.
AB is the diameter of the circle with centre O. OD is parallel to BC and ∠ AOD = 60° ; calculate the numerical values of: ∠ ADC

Prove that the line segment joining the midpoints of two equal chords of a circle subtends equal angles with the chord.
In a circle with center O, chords AB and CD intersect inside the circumference at E. Prove that ∠ AOC + ∠ BOD = 2∠ AEC.
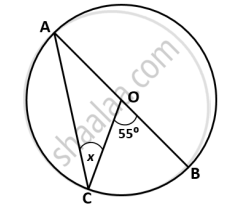
In the given figure, AB is a diameter of the circle with centre ‘O’. If ∠COB = 55⁰ then the value of x is: