Advertisements
Advertisements
प्रश्न
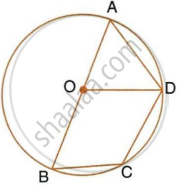
AB is the diameter of the circle with centre O. OD is parallel to BC and ∠AOD = 60°. Calculate the numerical values of :
- ∠ABD,
- ∠DBC,
- ∠ADC.
उत्तर
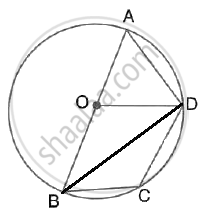
Join BD.
i. `∠ABD = 1/2 ∠AOD = 1/2xx 60^circ = 30^circ`
(Angle at the first is double the angle at the circumference subtended by the same chord)
ii. ∠BDA = 90°
(Angle in a semicircle)
Also, ΔOAD is equilateral (∵ ∠OAD = 60°)
∴ ∠ODB = 90° – ∠ODA
= 90° – 60°
= 30°
Also, OD || BC
∴ ∠DBC = ∠ODB = 30° (Alternate angles)
iii. ∠ABC = ∠ABD + ∠DBC
= 30° + 30°
= 60°
In cyclic quadrilateral ABCD,
∠ADC = 180° – ∠ABC
= 180° – 60°
= 120°
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
APPEARS IN
संबंधित प्रश्न
In the following figure, O is the centre of the circle, ∠AOB = 60° and ∠BDC = 100°. Find ∠OBC.

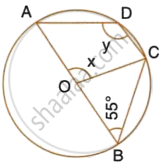
In the given figure, O is the centre of the circle and ∠ABC = 55°. Calculate the values of x and y.
In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find: ∠CAB
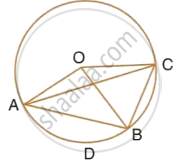
In the given figure, BD is a side of a regular hexagon, DC is a side of a regular pentagon and AD is a diameter.
Calculate :
- ∠ADC,
- ∠BDA,
- ∠ABC,
- ∠AEC.

The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given that ∠APB = a°.
Calculate, in terms of a°, the value of : ∠ACB,
Give reasons for your answers clearly.
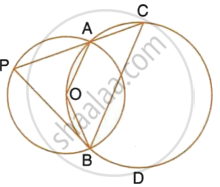
The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given that ∠APB = a°.
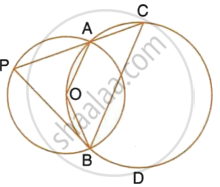
Calculate, in terms of a°, the value of : ∠ADB.
Give reasons for your answers clearly.
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠ CAB = xo ; find (in terms of x) the values of: ∠ DOC.
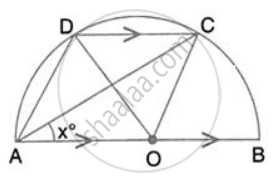
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠ CAB = xo ; find (in terms of x) the values of: ∠ ADC.
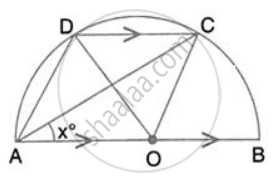
In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find : ∠ACB

If O is the circumcentre of a Δ ABC and OD ⊥ BC, prove that ∠ BOD = ∠A.
