Advertisements
Advertisements
प्रश्न
In a circle with center O, chords AB and CD intersect inside the circumference at E. Prove that ∠ AOC + ∠ BOD = 2∠ AEC.
उत्तर
Consider arc AC of the circle with the centre of at O.
Clearly, Arc AC subtends ∠ AOC at the centre and ∠ ABC at the remaining part of the circle.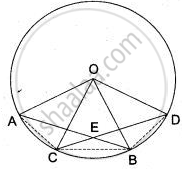
∴ ∠ AOC = 2 ∠ ABC ....(i)
Similarly, arc BD Subtends ∠ BOD at the centre and ∠ BCD at the remaining part of the circle.
∴ ∠ BOD = 2 ∠ BCD ....(ii)
Adding (i) and (ii), we get
∠ AOC + ∠ BOD = 2 (∠ ABC + ∠ BCD)
⇒ ∠ AOC + ∠ BOD = 2 ∠ AEC ...( ∵∠ AEC is the exterior angle and ∠ ABC and ∠BCD are other interior angles of Δ BEF ∴ ∠ ABC + ∠ BCD = ∠ AEC)
Hence proved.
APPEARS IN
संबंधित प्रश्न
In the figure, O is the centre of the circle, ∠AOE = 150°, ∠DAO = 51°. Calculate the sizes of the angles CEB and OCE.

In the given figure, AE is the diameter of the circle. Write down the numerical value of ∠ABC + ∠CDE. Give reasons for your answer.
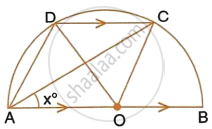
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠CAB = x°; find (in terms of x) the values of :
- ∠COB,
- ∠DOC,
- ∠DAC,
- ∠ADC.
In the given diagram, chord AB = chord BC.
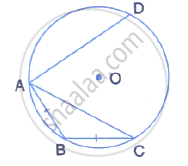
(i) what is the relation between arcs AB and BC?
(ii) what is the relation between ∠AOB and ∠BOC?
(iii) If arc AD is greater than arc ABC, then what is the relation between chords AD and AC?
(iv) If ∠AOB = 50°, find the measure of angle BAC.
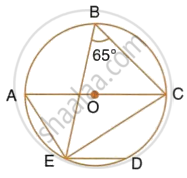
In the given figure, chord ED is parallel to diameter AC of the circle. Given ∠CBE = 65°, calculate ∠DEC.
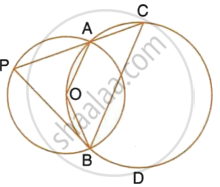
The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given that ∠APB = a°.
Calculate, in terms of a°, the value of : ∠ACB,
Give reasons for your answers clearly.
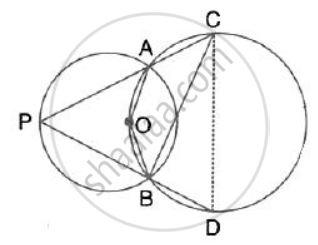
AB is the diameter of the circle with centre O. OD is parallel to BC and ∠ AOD = 60° ; calculate the numerical values of: ∠ DBC

In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find : ∠ABD
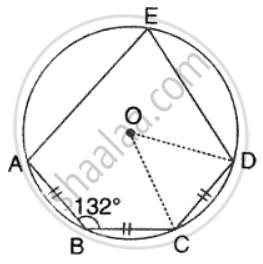
In the given figure, AB = BC = CD and ∠ABC = 132° . Calcualte: ∠ COD.
Prove that the line segment joining the midpoints of two equal chords of a circle subtends equal angles with the chord.
