Advertisements
Advertisements
प्रश्न
In a circle with center O, chords AB and CD intersect inside the circumference at E. Prove that ∠ AOC + ∠ BOD = 2∠ AEC.
उत्तर
Consider arc AC of the circle with the centre of at O.
Clearly, Arc AC subtends ∠ AOC at the centre and ∠ ABC at the remaining part of the circle.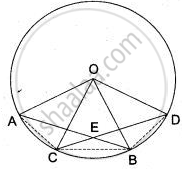
∴ ∠ AOC = 2 ∠ ABC ....(i)
Similarly, arc BD Subtends ∠ BOD at the centre and ∠ BCD at the remaining part of the circle.
∴ ∠ BOD = 2 ∠ BCD ....(ii)
Adding (i) and (ii), we get
∠ AOC + ∠ BOD = 2 (∠ ABC + ∠ BCD)
⇒ ∠ AOC + ∠ BOD = 2 ∠ AEC ...( ∵∠ AEC is the exterior angle and ∠ ABC and ∠BCD are other interior angles of Δ BEF ∴ ∠ ABC + ∠ BCD = ∠ AEC)
Hence proved.
APPEARS IN
संबंधित प्रश्न
In the figure given below, AB is diameter of the circle whose centre is O. given that: ∠ECD =
∠EDC = 32°. Show that ∠COF = ∠CEF.
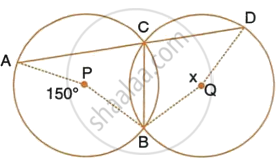
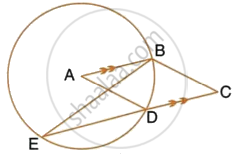
In the figure, given below, P and Q are the centres of two circles intersecting at B and C. ACD is a straight line. Calculate the numerical value of x .
In the given figure, A is the centre of the circle, ABCD is a parallelogram and CDE is a straight line. Prove that : ∠BCD = 2∠ABE.
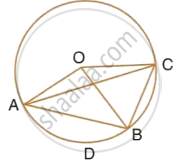
In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find: ∠CAB
The figure shows a circle with centre O. AB is the side of regular pentagon and AC is the side of regular hexagon. Find the angles of triangle ABC.

In the given figure, AOB is a diameter and DC is parallel to AB. If ∠ CAB = xo ; find (in terms of x) the values of: ∠ DAC
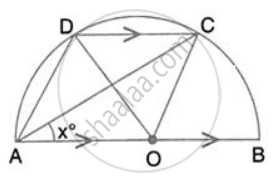
AB is the diameter of the circle with centre O. OD is parallel to BC and ∠ AOD = 60° ; calculate the numerical values of: ∠ ADC

In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find : ∠ABD
Prove that the line segment joining the midpoints of two equal chords of a circle subtends equal angles with the chord.
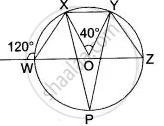
In the figure alongside O is the centre of circle ∠ XOY = 40°, ∠ TWX = 40° and XY is parallel to TZ.
Find: (i) ∠ XZY, (ii) ∠ YXZ (iii) ∠ TZY.