Advertisements
Advertisements
Question
In a circle with center O, chords AB and CD intersect inside the circumference at E. Prove that ∠ AOC + ∠ BOD = 2∠ AEC.
Solution
Consider arc AC of the circle with the centre of at O.
Clearly, Arc AC subtends ∠ AOC at the centre and ∠ ABC at the remaining part of the circle.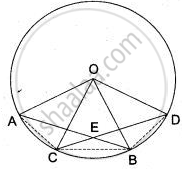
∴ ∠ AOC = 2 ∠ ABC ....(i)
Similarly, arc BD Subtends ∠ BOD at the centre and ∠ BCD at the remaining part of the circle.
∴ ∠ BOD = 2 ∠ BCD ....(ii)
Adding (i) and (ii), we get
∠ AOC + ∠ BOD = 2 (∠ ABC + ∠ BCD)
⇒ ∠ AOC + ∠ BOD = 2 ∠ AEC ...( ∵∠ AEC is the exterior angle and ∠ ABC and ∠BCD are other interior angles of Δ BEF ∴ ∠ ABC + ∠ BCD = ∠ AEC)
Hence proved.
APPEARS IN
RELATED QUESTIONS
The figure given below, shows a Circle with centre O.
Given: ∠AOC = a and ∠ABC = b.
Find the relationship between a and b

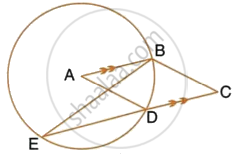
In the given figure, A is the centre of the circle, ABCD is a parallelogram and CDE is a straight line. Prove that : ∠BCD = 2∠ABE.
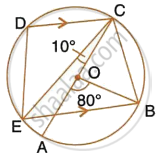
In the given figure, AC is the diameter of the circle with centre O. CD and BE are parallel. Angle ∠AOB = 80° and ∠ACE = 10°.
Calculate:
- Angle BEC,
- Angle BCD,
- Angle CED.
In the given figure, AE is the diameter of the circle. Write down the numerical value of ∠ABC + ∠CDE. Give reasons for your answer.
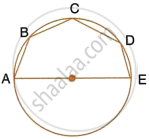
In a regular pentagon ABCDE, inscribed in a circle; find ratio between angle EDA and angle ADC.
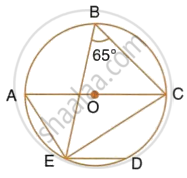
In the given figure, chord ED is parallel to diameter AC of the circle. Given ∠CBE = 65°, calculate ∠DEC.
In the given figure, AB is the diameter of the circle with centre O.
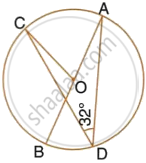
If ∠ADC = 32°, find angle BOC.
In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find : ∠ACB

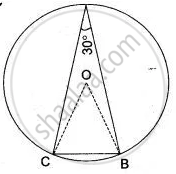
In the given Figure, ABC is a triangle in which ∠BAC = 30°. Show that BC is the radius of the circumcircle of A ABC, whose center is O.
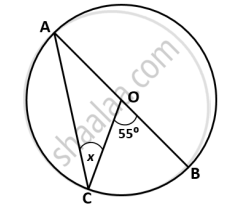
In the given figure, AB is a diameter of the circle with centre ‘O’. If ∠COB = 55⁰ then the value of x is: