Advertisements
Advertisements
Question
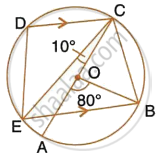
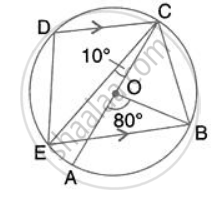
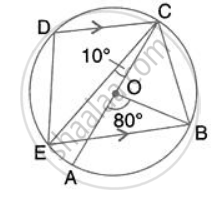
In the given figure, AC is the diameter of the circle with centre O. CD and BE are parallel. Angle ∠AOB = 80° and ∠ACE = 10°.
Calculate:
- Angle BEC,
- Angle BCD,
- Angle CED.
Solution
i. ∠BOC = 180° – 80° = 100° (Straight line)
And ∠BOC = 2∠BEC
(Angle at the centre is double the angle at the circumference subtended by the same chord)
`=> ∠BEC = (100^circ)/2 = 50^circ`
ii. DC || EB
∴ DCE = ∠BEC = 50° (Alternate angles)
∴ ∠AOB = 80°
`=> ∠ACB = 1/2 ∠AOB = 40^circ`
Angle at the center is double the angle at the circumference subtended by the same chord)
We have,
∠BCD = ∠ACB + ∠ACE + ∠DCE
= 40° + 10° + 50°
= 100°
iii. ∠BED = 180° – ∠BCD
= 180° – 100°
= 80°
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
`=>` ∠CED + 50° = 80°
`=>` ∠CED = 30°
APPEARS IN
RELATED QUESTIONS
In the given figure, AE is the diameter of the circle. Write down the numerical value of ∠ABC + ∠CDE. Give reasons for your answer.
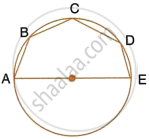
In the given figure, AB = BC = CD and ∠ABC = 132°.
Calcualte:
- ∠AEB,
- ∠AED,
- ∠COD.

In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find: ∠CAB
In the given figure, AC is the diameter of circle, centre O. CD and BE are parallel. Angle AOB = 80o and angle ACE = 10o. Calculate : Angle BCD
In the given figure, AC is the diameter of circle, centre O. CD and BE are parallel. Angle AOB = 80o and angle ACE = 10o. Calculate: Angle CED.
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠ CAB = xo ; find (in terms of x) the values of: ∠ DOC.
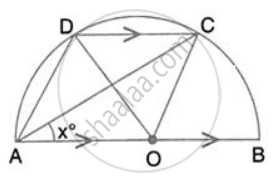
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠ CAB = xo ; find (in terms of x) the values of: ∠ ADC.
Prove that the line segment joining the midpoints of two equal chords of a circle subtends equal angles with the chord.
In a circle with center O, chords AB and CD intersect inside the circumference at E. Prove that ∠ AOC + ∠ BOD = 2∠ AEC.
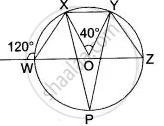
In the figure alongside O is the centre of circle ∠ XOY = 40°, ∠ TWX = 40° and XY is parallel to TZ.
Find: (i) ∠ XZY, (ii) ∠ YXZ (iii) ∠ TZY.