Advertisements
Advertisements
Question
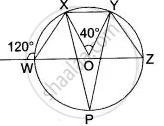
In the figure alongside O is the centre of circle ∠ XOY = 40°, ∠ TWX = 40° and XY is parallel to TZ.
Find: (i) ∠ XZY, (ii) ∠ YXZ (iii) ∠ TZY.
Solution
Construction: Take a point P on the circumference of the circle. Join XP and YP.
Determination of Amgles:
(i) ∠ XOY = 2∠ XPY ....( Angle subtended by an arc of a circle at the centre is twice the angle subtended by that arc at any point on the circumference of the circle)
⇒ 40° = 2 ∠ XPY ....( ∵ ∠ XOY = 40° )
⇒ ∠ XPY = `(40°)/2` = 20°
⇒ ∠ XPY = 20° ...( ∵ ∠ XPY = ∠ XZY )
Angles in a same segment of a circle are equal.
(ii) ∠ XWT + ∠ XWZ = 180° ...(Linear Pair Axiom)
⇒ 120° + ∠ XWZ = 180°
⇒ ∠ XWZ = 180° - 120° = 60°
∠ XWZ + ∠ XYZ = 180° ....(Opposite angles of a cyclic quadrilateral are supplementary)
⇒ 60° + ∠ XYZ = 180°
⇒ ∠ XYZ = 180° - 60° = 120°
∴ In ΔXYZ,
∠ YXZ + 120° + 20° = 180°
⇒ ∠ YXZ + 140° = 180°
⇒ ∠ YXZ = 180° - 140° = 40°
(iii) ∵ XY || TZ and transversal YZ intersects then
⇒ ∠ XYZ + ∠ TZY = 180° ....(Sum of the consecutive interior angles is 180° )
⇒ 120° + ∠ TZY = 180°
⇒ ∠ TZY = 180° - 120° = 60°
APPEARS IN
RELATED QUESTIONS
In the following figure, O is the centre of the circle, ∠AOB = 60° and ∠BDC = 100°. Find ∠OBC.

AB is the diameter of the circle with centre O. OD is parallel to BC and ∠AOD = 60°. Calculate the numerical values of :
- ∠ABD,
- ∠DBC,
- ∠ADC.
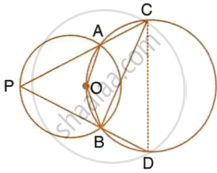
In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find :
- ∠AOB,
- ∠ACB,
- ∠ABD,
- ∠ADB.
In a regular pentagon ABCDE, inscribed in a circle; find ratio between angle EDA and angle ADC.
In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find: ∠CAB
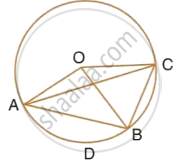
The figure shows a circle with centre O. AB is the side of regular pentagon and AC is the side of regular hexagon. Find the angles of triangle ABC.

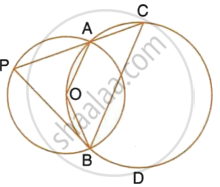
The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given that ∠APB = a°.
Calculate, in terms of a°, the value of : ∠ADB.
Give reasons for your answers clearly.
In a circle with center O, chords AB and CD intersect inside the circumference at E. Prove that ∠ AOC + ∠ BOD = 2∠ AEC.
C is a point on the minor arc AB of the circle, with centre O. Given ∠ACB = x° and ∠AOB = y° express y in terms of x. Calculate x, if ACBO is a parallelogram.
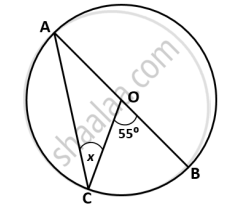
In the given figure, AB is a diameter of the circle with centre ‘O’. If ∠COB = 55⁰ then the value of x is: