Advertisements
Advertisements
Question
The figure shows a circle with centre O. AB is the side of regular pentagon and AC is the side of regular hexagon. Find the angles of triangle ABC.

Solution
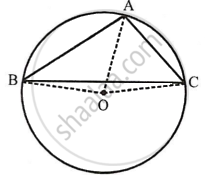
Join OA, OB and OC
Since AB is the side of a regular pentagon,
`∠AOB = 360^circ/5 = 72^circ`
Again AC is the side of a regular hexagon,
`∠AOC = 360^circ/6 = 60^circ`
But ∠AOB + ∠AOC + ∠BOC = 360° ...[Angles at a point]
`=>` 72° + 60° + ∠BOC = 360°
`=>` 132° + ∠BOC = 360°
`=>` ∠BOC = 360° – 132°
`=>` ∠BOC = 228°
Now, Arc BC subtends ∠BOC at the centre and ∠BAC at the remaining part of the circle.
`=> ∠BAC = 1/2 ∠BOC`
`=> ∠BAC = 1/2 xx 228^circ = 114^circ`
Similarly, we can prove that
`=> ∠ABC = 1 /2∠AOC`
`=> ∠ABC = 1 /2 xx 60^circ = 30^circ`
And
`=> ∠ACB = 1/2 AOB`
`=> ∠ACB = 1/2 xx 72^circ = 36^circ `
Thus, angles of the triangle are, 114°, 30° and 36°
APPEARS IN
RELATED QUESTIONS
In the figure given below, AB is diameter of the circle whose centre is O. given that: ∠ECD =
∠EDC = 32°. Show that ∠COF = ∠CEF.
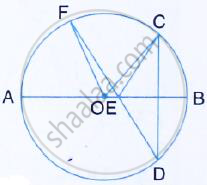
AB is the diameter of the circle with centre O. OD is parallel to BC and ∠AOD = 60°. Calculate the numerical values of :
- ∠ABD,
- ∠DBC,
- ∠ADC.
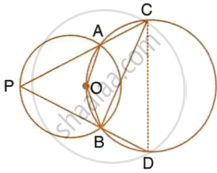
In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find :
- ∠AOB,
- ∠ACB,
- ∠ABD,
- ∠ADB.
In the given diagram, chord AB = chord BC.
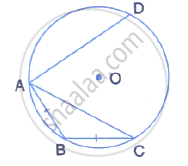
(i) what is the relation between arcs AB and BC?
(ii) what is the relation between ∠AOB and ∠BOC?
(iii) If arc AD is greater than arc ABC, then what is the relation between chords AD and AC?
(iv) If ∠AOB = 50°, find the measure of angle BAC.
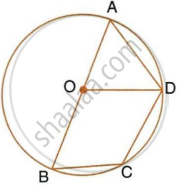
In the given figure, BD is a side of a regular hexagon, DC is a side of a regular pentagon and AD is a diameter.
Calculate :
- ∠ADC,
- ∠BDA,
- ∠ABC,
- ∠AEC.

In the given figure, chord ED is parallel to diameter AC of the circle. Given ∠CBE = 65°, calculate ∠DEC.
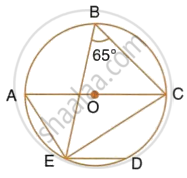
In the given figure, AB = BC = CD and ∠ABC = 132° . Calcualte: ∠AED

In a circle with center O, chords AB and CD intersect inside the circumference at E. Prove that ∠ AOC + ∠ BOD = 2∠ AEC.
In the given Figure, ABC is a triangle in which ∠BAC = 30°. Show that BC is the radius of the circumcircle of A ABC, whose center is O.
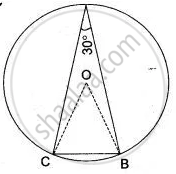
If O is the circumcentre of a Δ ABC and OD ⊥ BC, prove that ∠ BOD = ∠A.
