Advertisements
Advertisements
Question
In the given figure, AC is a diameter of circle, centre O. Chord BD is perpendicular to AC. Write down the angles p, q and r in terms of x.

Solution

∵ Arc subtends ∠AOB at the centre and ∠ACB at the remaining part of the circle.
∴ ∠AOB = 2∠ACB
`\implies` x = 2q
`\implies q = x/2`
But ∠ADB and ∠ACB are in the same segment
∴ ∠ADB = ∠ACB = q
Now in ΔAED.
p + q + 90° = 180° ...(Sum of angles of a Δ)
p + q = 90°
p = 90° – q
`p = 90^circ - x/2`
∵ Arc BC subtends ∠BOC at the centre and ∠ADC at the remaining part of the circle
∴ ∠BOC = 2∠BDC = 2r
∴ `r = 1/2 ∠BOC = 1/2 (180^circ - x)`
∵ (∠AOB + ∠BOC = 180°)
∴ `r = 90^circ - 1/2 x`
= `90^circ - x/2`
APPEARS IN
RELATED QUESTIONS
The radius of a circle is 17.0 cm and the length of perpendicular drawn from its centre to a chord is 8.0 cm. Calculate the length of the chord.
In Δ ABC, the perpendicular from vertices A and B on their opposite sides meet (when produced) the circumcircle of the triangle at points D and E respectively. Prove that: arc CD = arc CE
Two chords of lengths 10cm and 24cm are drawn parallel o each other in a circle. If they are on the same side of the centre and the distance between them is 17cm, find the radius of the circle.
In following figure , AB , a chord of the circle is of length 18 cm. It is perpendicularly bisected at M by PQ.
Two parallel chords are drawn in a circle of diameter 30.0 cm. The length of one chord is 24.0 cm and the distance between the two chords is 21.0 cm;
find the length of another chord.
In a circle of radius 17 cm, two parallel chords of lengths 30 cm and 16 cm are drawn. Find the distance between the chords,
if both the chords are:
(i) on the opposite sides of the centre;
(ii) on the same side of the centre.
In the following figure, AD is a straight line, OP ⊥ AD and O is the centre of both circles. If OA = 34cm, OB = 20 cm and OP = 16 cm;
find the length of AB.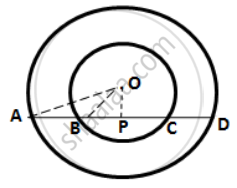
The figure shows two concentric circles and AD is a chord of a larger circle.
Prove that: AB = CD.
In the given figure, OD is perpendicular to the chord AB of a circle whose center is O. If BC is a diameter, show that CA = 2 OD.
The radius of a circle is 13 cm and the length of one of its chord is 10 cm. Find the distance of the chord from the centre.
