Advertisements
Advertisements
Question
Two chords of lengths 10cm and 24cm are drawn parallel o each other in a circle. If they are on the same side of the centre and the distance between them is 17cm, find the radius of the circle.
Solution
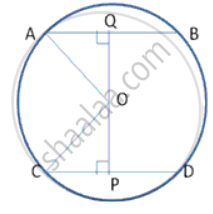
CP = PO = 12cm
Let OA = OC = r (say)
Also, let OQ = x, ∴ OP = 17 - x
In right Δ OPC,
By Pythagoras theorem,
OC2 = OP2 + PC2
r2 = (17- x)2 + 122 ----( 1 )
Similarly, In Δ OQA,
OA2 = AQ2 + QO2
r2 = 52 + x2 ----(2}
From (1) and { 2}
( 17 - x)2 + 122 = 52 + x2
289 - 34 x + 144 - 25 = 0
34x = 408
x = 12
From {2}
r2 = 52 + 122
25+ 144= 169
r = 13
The radius of the circle is 13cm .
APPEARS IN
RELATED QUESTIONS
AB and CD are two parallel chords of a circle such that AB = 24 cm and CD = 10 cm. If the
radius of the circle is 13 cm. find the distance between the two chords.

The figure shows two concentric circles and AD is a chord of larger circle.
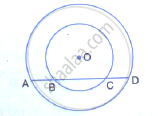
Prove that: AB = CD
In the given figure, AC is a diameter of circle, centre O. Chord BD is perpendicular to AC. Write down the angles p, q and r in terms of x.

In the following figure, a circle is inscribed in the quadrilateral ABCD.

If BC = 38 cm, QB = 27 cm, DC = 25 cm and that AD is perpendicular to DC, find the radius of the circle.
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other.
If ∠MAD = x and ∠BAC = y : express ∠ABD in terms of y.

A chord of length 24 cm is at a distance of 5 cm from the center of the circle. Find the length of the chord of the same circle which is at a distance of 12 cm from the center.
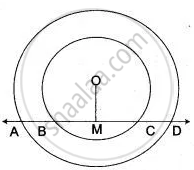
In the given figure, l is a line intersecting the two concentric circles, whose common center is O, at the points A, B, C, and D. Show that AB = CD.
The radius of a circle is 13 cm and the length of one of its chord is 10 cm. Find the distance of the chord from the centre.
AB is a diameter of a circle with centre C = (- 2, 5). If A = (3, – 7). Find
(i) the length of radius AC
(ii) the coordinates of B.
In the figure given below, O is the centre of the circle. AB and CD are two chords of the circle. OM is perpendicular to AB and ON is perpendicular to CD.

AB = 24 cm, OM = 5 cm, ON = 12 cm. Find the
(i) radius of the circle
(ii) length of chord CD.
