Advertisements
Advertisements
Question
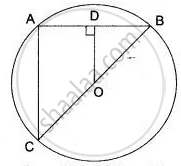
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other.
If ∠MAD = x and ∠BAC = y : express ∠ABD in terms of y.

Solution
In the figure, M is the centre of the circle.
Chords AB and CD are perpendicular to each other at L.
∠MAD = x and ∠BAC = y
∴ Arc AD ∠AMDat the centre and ∠ABD at the remaining
(Angle in the same segment)
(Angle at the centre is double the angle at the circumference subtended by the same chord)
⇒ ∠AMD = 2∠ABD
⇒ ∠ABD = `1/2 (180° - 2x )`
⇒∠ABD = 90° - x
AB ⊥ CD, ∠ALC = 90°
In ∆ALC,
∴ ∠LAC +∠LCA = 90°
⇒ ∠BAC + ∠DAC = 90°
⇒ y + ∠DAC = 90°
∴ ∠DAC = 90° - y
We have, ∠DAC = ∠ABD [Angles in the same segment]
∴ ∠ABD = 90° - y
APPEARS IN
RELATED QUESTIONS
AB and CD are two parallel chords of a circle such that AB = 24 cm and CD = 10 cm. If the
radius of the circle is 13 cm. find the distance between the two chords.

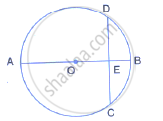
The figure given below, shows a circle with centre O in which diameter AB bisects the chord CD at point E. If CE = ED = 8 cm and EB = 4cm, find the radius of the circle.
The figure shows two concentric circles and AD is a chord of larger circle.
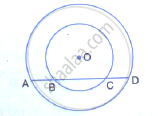
Prove that: AB = CD
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other. If ∠MAD = x and ∠BAC = y :
- express ∠AMD in terms of x.
- express ∠ABD in terms of y.
- prove that : x = y.

Two chords of lengths 10cm and 24cm are drawn parallel o each other in a circle. If they are on the same side of the centre and the distance between them is 17cm, find the radius of the circle.
A chord of length 6 cm is drawn in a circle of radius 5 cm.
Calculate its distance from the center of the circle.
M and N are the mid-points of two equal chords AB and CD respectively of a circle with center O.
Prove that: (i) ∠BMN = ∠DNM
(ii) ∠AMN = ∠CNM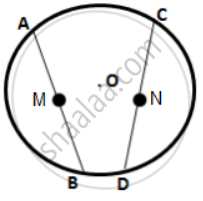
The radius of a circle is 13 cm and the length of one of its chords is 24 cm.
Find the distance of the chord from the center.
In the given figure, OD is perpendicular to the chord AB of a circle whose center is O. If BC is a diameter, show that CA = 2 OD.
AB and CD are two parallel chords of a circle such that AB = 10 cm and CD = 24 cm. If the chords are on the opposite sides of the centre and the distance between them is 17 cm, find the radius of the circle.
