Advertisements
Advertisements
Question
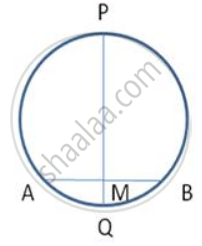
In following figure , AB , a chord of the circle is of length 18 cm. It is perpendicularly bisected at M by PQ.
Solution
Given : AB = 18 cm , MQ = 3 cm
To find : PQ

OQ = OA = r cm (say)
∴ OM = OQ = MQ = (r - 3) cm
AM = MB = 9 cm (PQ ⊥ AB)
In right Δ OMA ,
OM2 + MA2 = OA2
⇒ (r - 3)2 + 92 = r2
⇒ r2 - 6r + 9 + 81 = r2
⇒ 6r = 90
⇒ r = 15 cm
PQ = 2r
(Perpendicular bisector of a chord passes through the centre of the circe)
PQ = 2(15)
PQ = 30 cm
APPEARS IN
RELATED QUESTIONS
The radius of a circle is 17.0 cm and the length of perpendicular drawn from its centre to a chord is 8.0 cm. Calculate the length of the chord.
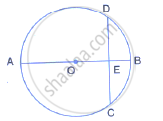
The figure given below, shows a circle with centre O in which diameter AB bisects the chord CD at point E. If CE = ED = 8 cm and EB = 4cm, find the radius of the circle.
In Δ ABC, the perpendicular from vertices A and B on their opposite sides meet (when produced) the circumcircle of the triangle at points D and E respectively. Prove that: arc CD = arc CE
In the following figure, a circle is inscribed in the quadrilateral ABCD.

If BC = 38 cm, QB = 27 cm, DC = 25 cm and that AD is perpendicular to DC, find the radius of the circle.
PQ and QR are two equal chords of a circle. A diameter of the circle is drawn through Q . Prove that the diameter bisects ∠ PQR.
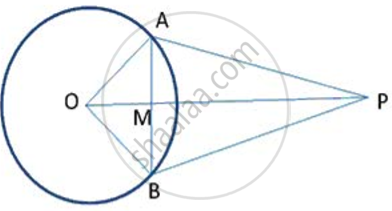
From a point P outside a circle, with centre O. tangents PA and PB are drawn as following fig., Prove that ∠ AOP = ∠ BOP and OP is the perpendicular bisector of AB.
In the following figure, the line ABCD is perpendicular to PQ; where P and Q are the centers of the circles.
Show that:
(i) AB = CD ;
(ii) AC = BD.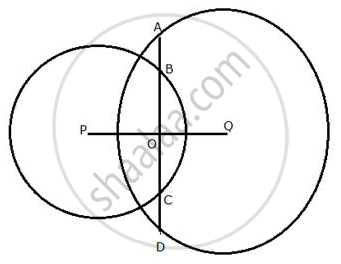
In the given figure, OD is perpendicular to the chord AB of a circle whose center is O. If BC is a diameter, show that CA = 2 OD.
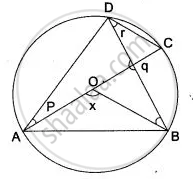
In the figure, AC is the diameter of circle, centre O. Chord BD is perpendicular to AC. Write down the angles p, q, r in term of x.
AB, CD are parallel chords of a circle 7 cm apart. If AB = 6 cm, CD = 8 cm, find the radius of the circle.
