Advertisements
Advertisements
Question
AB, CD are parallel chords of a circle 7 cm apart. If AB = 6 cm, CD = 8 cm, find the radius of the circle.
Solution
Let O be the centre of the circle OM and ON are perpendiculars on AB and CD.
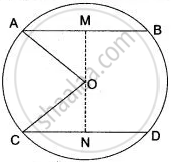
MON is one straight line.
Here
AM = `1/2`AB = 3 cm
CN = `1/2`CD = 4 cm
Let, ON = x cm and radius OA = OC = r cm
From right angled triangle OCN,
ON2 = OC2 - CN2 ...(By Pythagoras Theorem)
x2 = r2 - 16 ...(1)
From right-angled triangle OAM,
OM2 = OA2 - AM2 ...(By Pythagoras Theorem)
(7 - x)2 = r2 - 9 ...(2)
From (1) and (2),
(7 - x)2 - x2 = 7
49 + x2 - 14x - x2 = 7
14x = 42
x = 3
r2 = x2 + 16 ....(From(1))
r2 = 9 + 16 = 25
r = 5 cm
Hence, the radius of the circle is 5 cm.
APPEARS IN
RELATED QUESTIONS
A chord CD of a circle whose centre is O, is bisected at P by a diameter AB.
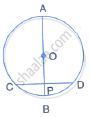
Given OA = OB = 15 cm and OP = 9 cm. calculate the length of:
(i) CD (ii) AD (iii) CB
In the following figure, the line ABCD is perpendicular to PQ; where P and Q are the centres of
the circles. Show that:
(i) AB = CD,
(ii) AC = BD.

In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other. If ∠MAD = x and ∠BAC = y :
- express ∠AMD in terms of x.
- express ∠ABD in terms of y.
- prove that : x = y.

In Δ ABC, the perpendicular from vertices A and B on their opposite sides meet (when produced) the circumcircle of the triangle at points D and E respectively. Prove that: arc CD = arc CE
In the following figure, a circle is inscribed in the quadrilateral ABCD.

If BC = 38 cm, QB = 27 cm, DC = 25 cm and that AD is perpendicular to DC, find the radius of the circle.
PQ and QR are two equal chords of a circle. A diameter of the circle is drawn through Q . Prove that the diameter bisects ∠ PQR.
A chord of length 6 cm is drawn in a circle of radius 5 cm.
Calculate its distance from the center of the circle.
In the following figure, the line ABCD is perpendicular to PQ; where P and Q are the centers of the circles.
Show that:
(i) AB = CD ;
(ii) AC = BD.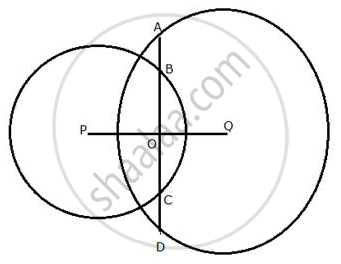
In a circle of radius 10 cm, AB and CD are two parallel chords of lengths 16 cm and 12 cm respectively.
Calculate the distance between the chords, if they are on:
(i) the same side of the center.
(ii) the opposite sides of the center.
AB is a diameter of a circle with centre O and radius OD is perpendicular to AB. If C is any point on arc DB, find ∠ BAD and ∠ ACD.
