Advertisements
Advertisements
Question
AB is a diameter of a circle with centre O and radius OD is perpendicular to AB. If C is any point on arc DB, find ∠ BAD and ∠ ACD.
Solution
Since, chord BD makes ∠BOD at the centre and ∠BAD at A.
∴ ∠BAD = `1/2`∠BOD
= `1/2` x 90°
= 45°
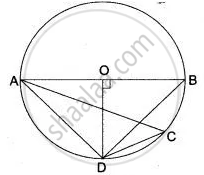
Similarly,
Chord AD makes ∠AOD at the centre and ∠ACD at C.
∴ ∠ACD = `1/2`∠AOD
= `1/2` x 90° = 45°
Thus, ∠BAD = ∠ACD = 45°.
APPEARS IN
RELATED QUESTIONS
The figure shows two concentric circles and AD is a chord of larger circle.
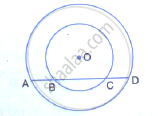
Prove that: AB = CD
In the following figure, the line ABCD is perpendicular to PQ; where P and Q are the centres of
the circles. Show that:
(i) AB = CD,
(ii) AC = BD.

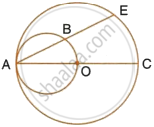
In the given figure, AC is a diameter of a circle, whose centre is O. A circle is described on AO as diameter. AE, a chord of the larger circle, intersects the smaller circle at B. Prove that : AB = BE.
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other.
If ∠MAD = x and ∠BAC = y : express ∠ABD in terms of y.

Two parallel chords are drawn in a circle of diameter 30.0 cm. The length of one chord is 24.0 cm and the distance between the two chords is 21.0 cm;
find the length of another chord.
In a circle of radius 17 cm, two parallel chords of lengths 30 cm and 16 cm are drawn. Find the distance between the chords,
if both the chords are:
(i) on the opposite sides of the centre;
(ii) on the same side of the centre.
A chord of length 24 cm is at a distance of 5 cm from the center of the circle. Find the length of the chord of the same circle which is at a distance of 12 cm from the center.
In the following figure, the line ABCD is perpendicular to PQ; where P and Q are the centers of the circles.
Show that:
(i) AB = CD ;
(ii) AC = BD.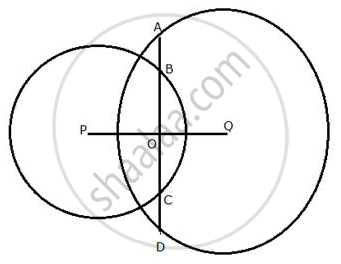
In a circle of radius 10 cm, AB and CD are two parallel chords of lengths 16 cm and 12 cm respectively.
Calculate the distance between the chords, if they are on:
(i) the same side of the center.
(ii) the opposite sides of the center.
Find the length of a chord which is at a distance of 5 cm from the centre of a circle of radius 13 cm.
