Advertisements
Advertisements
प्रश्न
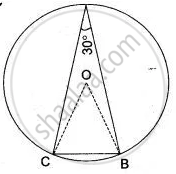
In the given Figure, ABC is a triangle in which ∠BAC = 30°. Show that BC is the radius of the circumcircle of A ABC, whose center is O.
उत्तर
Join OB and OC. Since the angle subtended by an arc of a circle at its center is twice the angle subtended by the same arc at a point on the circumference.
∴ ∠ BOC = 2 ∠ BAC
⇒ ∠ BOC = 2 x 30° = 60°
Now in Δ BOC, we have
OB = OC ...(Each equal to the radius of the circle)
⇒ ∠ OBC = ∠ OCB ...(∵ Angles opposite to equal sides of a triangle are equal)
But ∠ OBC + ∠ OCB + ∠ BOC = 180°
∴ 2 ∠ OBC + 60° = 180°
⇒ 2 ∠ OBC = 120°
⇒ ∠ OBC = 60°
Thus,
∠ OBC = ∠ OCB
∠ BOC = 60°
⇒ Triangle OBC is an equilateral
⇒ OB = BC ...(Showed)
⇒ BC is the radius of the circumcircle of Δ ABC.
Hence proved.
APPEARS IN
संबंधित प्रश्न
In the following figure, O is the centre of the circle, ∠AOB = 60° and ∠BDC = 100°. Find ∠OBC.

In the figure given below, AB is diameter of the circle whose centre is O. given that: ∠ECD =
∠EDC = 32°. Show that ∠COF = ∠CEF.
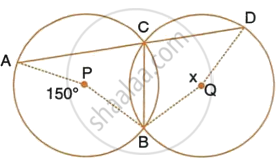
In the figure, given below, P and Q are the centres of two circles intersecting at B and C. ACD is a straight line. Calculate the numerical value of x .
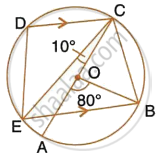
In the given figure, AC is the diameter of the circle with centre O. CD and BE are parallel. Angle ∠AOB = 80° and ∠ACE = 10°.
Calculate:
- Angle BEC,
- Angle BCD,
- Angle CED.
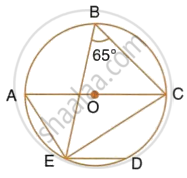
In the given figure, chord ED is parallel to diameter AC of the circle. Given ∠CBE = 65°, calculate ∠DEC.
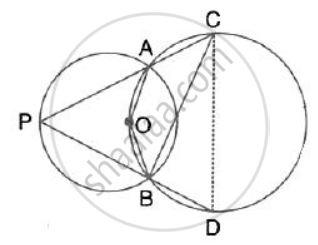
In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find : ∠ABD
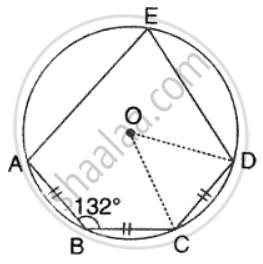
In the given figure, AB = BC = CD and ∠ABC = 132° . Calcualte: ∠ COD.
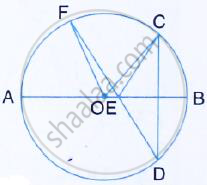
In a circle with center O, chords AB and CD intersect inside the circumference at E. Prove that ∠ AOC + ∠ BOD = 2∠ AEC.
If O is the circumcentre of a Δ ABC and OD ⊥ BC, prove that ∠ BOD = ∠A.
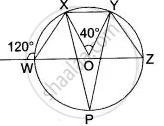
In the figure alongside O is the centre of circle ∠ XOY = 40°, ∠ TWX = 40° and XY is parallel to TZ.
Find: (i) ∠ XZY, (ii) ∠ YXZ (iii) ∠ TZY.